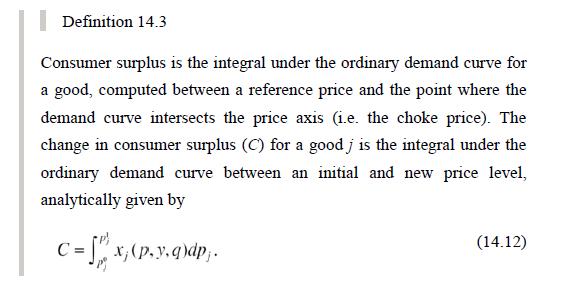
In section 14.2.2 we showed that for consumer surplus to be a unique, money-metric measure of utility change for price increases or decreases, the marginal utility of income must be constant with respect to the prices that change. We saw that this implies the income elasticities of demand for goods whose price change must be equal.
Use the Slutsky equation relationship for any two goods j and k whose prices change in the analysis to show that a constant marginal utility of income also implies equality of the ordinary demand cross price effects.

Data from section 14.2.2

Transcribed Image Text:

Equation (14.11) implies that EV and CV can be computed using the compensated demand curve, and as such they can be linked to a behavioral function for the good that has a price change. Typically, of course, it is ordinary rather than compensated demands that are estimated, and the integrals as given cannot be directly computed. A consequence is that consumer surplus has often been used in place of EV or CV, which is defined as follows.