Consider the model developed in Spiegel & Subrahmanyam [1558] and presented in Sect. 10.2. In this exercise,

Question:

Consider the model developed in Spiegel \& Subrahmanyam [1558] and presented in Sect. 10.2. In this exercise, following Spiegel \& Subrahmanyam [1558, Appendix A], we are going to prove Proposition 10.5.

(i) Assume that each informed trader \(k \in\{1, \ldots, K\}\) conjectures that

- every uninformed agent \(n \in\{1, \ldots, N\}\) demands a quantity \(\tilde{w}_{n}=\gamma \tilde{e}_{n}\) of the security, for some constant \(\gamma\) to be determined;

- every other informed trader \(l \in\{1, \ldots, K\} \backslash\{k\}\) demands a quantity \(\tilde{x}_{l}=\) \(\beta\left(\tilde{\varepsilon}+\tilde{\eta}_{l}\right)\) of the security, for some constant \(\beta\) to be determined;

- the market maker sets a price \(\tilde{p}\) according to the rule \[\tilde{p}=\bar{d}+\lambda\left(\sum_{k=1}^{K} \tilde{x}_{k}+\sum_{n=1}^{N} \tilde{w}_{n}\right)\]
for some constant \(\lambda\) to be determined.
Show that the optimal quantity \(\tilde{x}_{k}^{*}\) of the informed agent \(k \in\{1, \ldots, K\}\), conditionally on the observation of the private signal \(\tilde{\varepsilon}+\tilde{\eta}_{k}\), is given by \[\tilde{x}_{k}^{*}=\sigma_{\varepsilon}^{2} \frac{1-\lambda(K-1) \beta}{2 \lambda\left(\sigma_{\varepsilon}^{2}+\sigma_{\eta}^{2}\right)}\left(\tilde{\varepsilon}+\tilde{\eta}_{k}\right)\]
Deduce that, in equilibrium, the constant \(\beta\) must satisfy \[\begin{equation*}
\beta=\frac{\sigma_{\varepsilon}^{2}}{\lambda\left((1+K) \sigma_{\varepsilon}^{2}+2 \sigma_{\eta}^{2}\right)} \tag{10.32}
\end{equation*}\]
(ii) Consider then the expected utility maximization problem of each uninformed trader. Suppose that each uninformed trader \(n \in\{1, \ldots, N\}\) conjectures that - every informed trader \(k \in\{1, \ldots, K\}\) demands a quantity \(\tilde{x}_{k}=\beta\left(\tilde{\varepsilon}+\tilde{\eta}_{k}\right)\) of the security;
- each other uninformed agent \(m \in\{1, \ldots, N\} \backslash\{n\}\) demands a quantity \(\tilde{w}_{m}=\) \(\gamma \tilde{e}_{m}\) of the security, for some constant \(\gamma\) to be determined;
- the market maker sets a price \(\tilde{p}\) according to the rule \[\tilde{p}=\bar{d}+\lambda\left(\sum_{k=1}^{K} \tilde{x}_{k}+\sum_{n=1}^{N} \tilde{w}_{n}\right)\]
for some constant \(\lambda\) to be determined.
Show that the optimal quantity \(\tilde{w}_{n}^{*}\) demanded by the risk averse uninformed agent \(n \in\{1, \ldots, N\}\), conditionally on his endowment \(\tilde{e}_{n}\), is given by \[\begin{equation*}
\tilde{w}_{n}^{*}=-\frac{a \sigma_{\varepsilon}^{2}(1-K \lambda \beta) \tilde{e}_{n}}{2 \lambda+a\left(\sigma_{\varepsilon}^{2}(1-K \lambda \beta)^{2}+K \sigma_{\eta}^{2} \lambda^{2} \beta^{2}+\lambda^{2}(N-1) \gamma^{2} \sigma_{e}^{2}\right)} \tag{10.33}
\end{equation*}\]
Deduce that, in equilibrium, the constant \(\gamma\) must satisfy the equation \(a \lambda^{2} \gamma^{3}(N-1) \sigma_{e}^{2}+\gamma\left(a K \sigma_{\eta}^{2} \lambda^{2} \beta^{2}+a \sigma_{\varepsilon}^{2}(1-K \lambda \beta)^{2}+2 \lambda\right)+a \sigma_{\varepsilon}^{2}(1-K \lambda \beta)=0\).

(iii) By relying on the zero expected profits condition for the risk neutral market maker, together with the linear price setting rule, deduce that in equilibrium the parameters \(\gamma\) and \(\lambda\) satisfy the equation \[\begin{equation*}
K \sigma_{\varepsilon}^{4}\left(\sigma_{\varepsilon}^{2}+\sigma_{\eta}^{2}\right)=N \gamma^{2} \lambda^{2} \sigma_{e}^{2}\left((1+K) \sigma_{\varepsilon}^{2}+2 \sigma_{\eta}^{2}\right)^{2} \tag{10.35}
\end{equation*}\]
and, as a consequence, \(\gamma\) is given by \[\gamma=-\frac{\sqrt{K \sigma_{\varepsilon}^{4}\left(\sigma_{\varepsilon}^{2}+\sigma_{\eta}^{2}\right)}}{\sqrt{N \sigma_{e}^{2}} \lambda\left((1+K) \sigma_{\varepsilon}^{2}+2 \sigma_{\eta}^{2}\right)} .\]
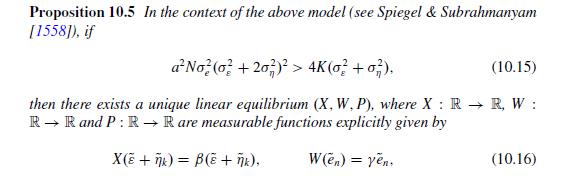
(iv) On the basis of the previous steps, deduce the result of Proposition 10.5.

Data From Proposition 10.5

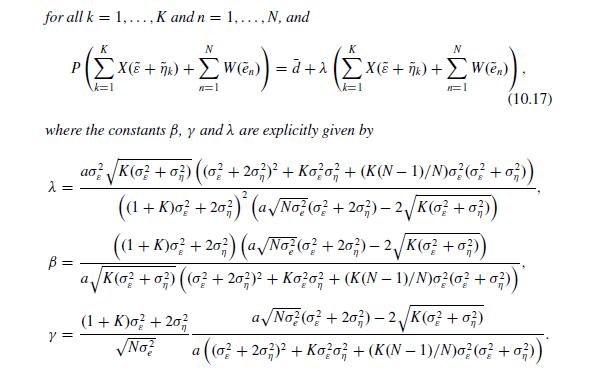
image text in transcribed