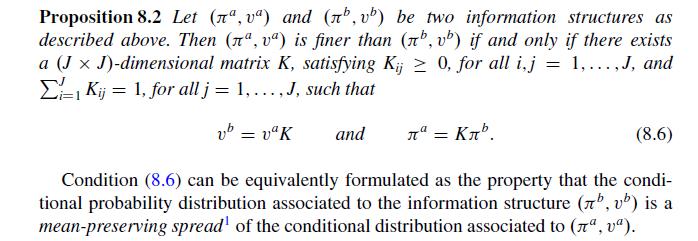
Let (left(pi^{a}, v^{a} ight)) and (left(pi^{b}, v^{b} ight)) be two information structures as described before Proposition 8.2.

Let \(\left(\pi^{a}, v^{a}\right)\) and \(\left(\pi^{b}, v^{b}\right)\) be two information structures as described before Proposition 8.2. Prove that the information structure \(\left(\pi^{a}, v^{a}\right)\) is finer than the information structure \(\left(\pi^{b}, v^{b}\right)\) if there exists a \((J \times J)\)-dimensional matrix \(K\), satisfying \(K_{i j} \geq 0\), for all \(i, j=1, \ldots, J\), and \(\sum_{i=1}^{J} K_{i j}=1\), for all \(j=1, \ldots, J\), such that condition (8.6) holds.

Data From Proposition 8.2

Transcribed Image Text:

Proposition 8.2 Let (,va) and (b, vb) be two information structures as described above. Then (a, va) is finer than (b, vb) if and only if there exists a (J x J)-dimensional matrix K, satisfying Kij > 0, for all i,j = 1,..., J, and Kij=1, for all j = 1,..., J, such that vb = vaK and = . (8.6) Condition (8.6) can be equivalently formulated as the property that the condi- tional probability distribution associated to the information structure (b, vb) is a mean-preserving spread of the conditional distribution associated to (", va).