The efficient market hypothesis (EMH), in its strong form, implies that asset returns over time are independent random variables. An investor does not believe the EMH and thinks that there is some degree of persistence in return. She estimates the following relationship between the random returns on an index at time periods \(t -\quad 1\) and \(t\) :

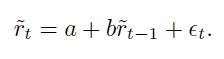
Note that, if \(b\) is positive, we have persistence in return. Assume that we know the parameters of the model, as well as the variance of the random shock term \({ }_{t}\) (whose expected value is zero). We use this statistical model to make portfolio choice on the basis of a mean-variance function

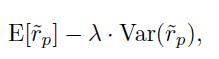
where \(r_{p}\) is the holding period return for a time period consisting of two consecutive time periods \(t\) and \(t+1\). In the portfolio, we mix the index and a risk-free asset with constant return \(r_{f}\) for each time period. Find the optimal portfolio weights.

Transcribed Image Text:

rt=a+brt-1 + Et.