A sufficient condition to produce positively weighted efficient portfolios is that the variance- covariance matrix be diagonal: That is, that ?_ij = 0, for i ? j. By continuity, positively weighted portfolios will result if the off-diagonal elements of the variance covariance matrix are suffi ciently small compared to the diagonal. Consider a transformation of the above matrix in which:

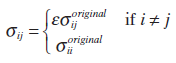
When ? = 1, this transformation will give the original variance-covariance matrix and when ? = 0, the transformation will give a fully diagonal matrix. For r = 10% find the maximum ? for which all portfolio weights are positive.

Transcribed Image Text:

original if i +j Oij original