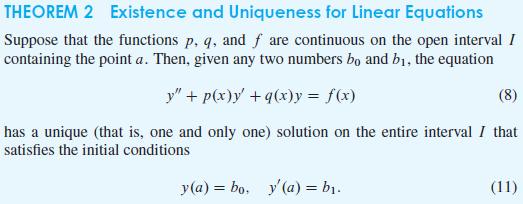
Given a mass m, a dashpot constant c, and a spring constant k, Theorem 2 of Section

Question:

Given a mass m, a dashpot constant c, and a spring constant k, Theorem 2 of Section 5.1 implies that the equation

has a unique solution for t ≧ 0 satisfying given initial conditions x (0) = x₀, x'(0) = v₀. Thus the future motion of an ideal mass-spring-dashpot system is completely determined by the differential equation and the initial conditions. Of course in a real physical system it is impossible to measure the parameters m, c, and k precisely. Problems 35 through 38 explore the resulting uncertainty in predicting the future behavior of a physical system.

Suppose that m = 1, c = 2, and k = 1 in Eq. (26). Show that the solution with x (0) = 0 and x' (0) = 1 is

x₁(t) = te^-t.