Problems 11 through 13 deal with the predator–prey system

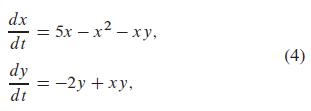
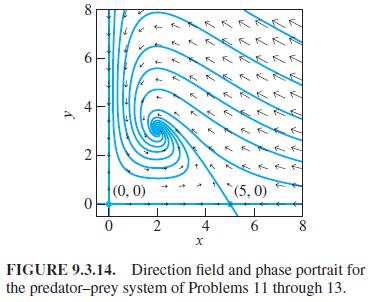
in which the prey population x (t) is logistic but the predator population y(t) would (in the absence of any prey) decline naturally. Problems 11 through 13 imply that the three critical points (0,0), (5,0), and (2,3) of the system in (4) are as shown in Fig. 9.3.14-with saddle points at the origin and on the positive x-axis, and with a spiral sink interior to the first quadrant. In each of these problems use a graphing calculator or computer system to construct a phase plane portrait for the linearization at the indicated critical point. Do your local portraits look consistent with Fig. 9.3.14?

Show that the coefficient matrix of the linearization x' = 5x, y' = -2y of (4) at (0,0) has the positive eigenvalue λ₁ = 5 and the negative eigenvalue λ₂ = -2. Hence (0,0) is a saddle point of the system in (4).

Transcribed Image Text:

dx dt dy dt = 5x-x²-xy, = -2y + xy, (4)