A truncated solid cone is of circular cross-section with a diameter that varies along its length according
Question:
A truncated solid cone is of circular cross-section with a diameter that varies along its length according to \(d(x)=a e^{x}\) where \(a=0.8 \mathrm{~m}\) and \(x\) is in meters. The cone has a length, \(L=\) \(1.8 \mathrm{~m}\), and a thermal conductivity, \(k=8 \mathrm{~W} / \mathrm{mK}\) and is shown in Figure P5.21. It also exhibits a uniform volumetric rate of heat generation, \(\dot{q}=1993 \mathrm{~W} / \mathrm{m}^{3}\). The sides of the cone are insulated and one end surface \((x=0)\) is held at \(T_{o}=300^{\circ} \mathrm{C}\) and has a heat flow rate, \(q=500 \mathrm{~W}\). Determine the temperature at the other end surface \((x=L)\) and the heat transfer rate there.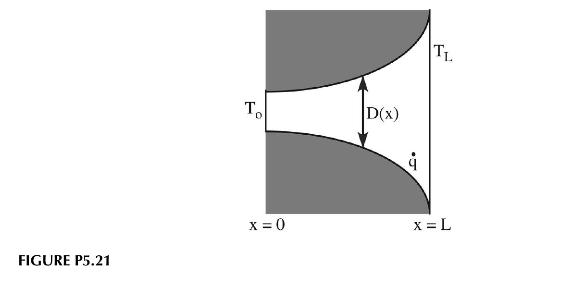
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Question Posted:





