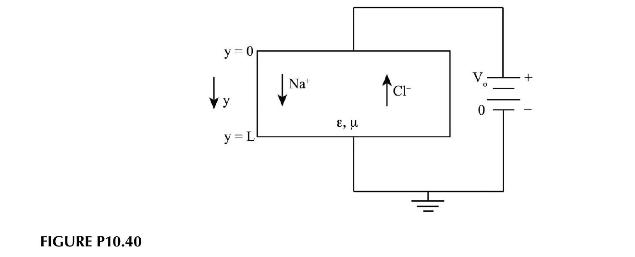
Consider the motion of sodium and chloride ions in a membrane under the influence of an externally applied electric field. The situation is shown in the Figure P10.40. Charge transport in the presence of an electric field obeys the following equations:

\[\begin{array}{ll}\frac{\partial^{2} V}{\partial y^{2}}=-\frac{q}{\varepsilon}(p-n) & V-\text { voltage } \\\frac{\partial p}{\partial t}=D \frac{\partial^{2} p}{\partial y^{2}}+\mu \frac{\partial}{\partial y}\left(p \frac{\partial V}{\partial y}\right) & q-\text { charge on electron } \\& p-\text { permittivity } \\\frac{\partial n}{\partial t}=D \frac{\partial^{2} n}{\partial y^{2}}-\mu \frac{\partial}{\partial y}\left(n \frac{\partial V}{\partial y}\right) & D-\text { diffusvity } \\& \mu-\text { mobility }\end{array}\]

a. Assuming steady state and that diffusion is negligible, derive one conservation of mass equation for the excess charge in the device.

b. Solve the equations for the voltage distribution and excess charge inside the medium.

c. Assuming the charge imbalance is known and is \(\Psi_{o}\), what boundary conditions would you impose on the system? Why?

d. What does your solution say about the relationship between the charge imbalance and the electric field \((E=-d V / d y)\) ?

Transcribed Image Text:

FIGURE P10.40 y=0 by y=L Na , 0