Often, we have thermo-capillary convection where one surface is free. The temperature gradient induces a change in
Question:
Often, we have thermo-capillary convection where one surface is free. The temperature gradient induces a change in surface tension that can drive the motion of the liquid. Thus, at the free surface there is a shear stress related to the temperature gradient.
\[y=L \quad \mu \frac{\partial v_{x}}{\partial y}=\frac{\partial \gamma}{\partial T} \quad \frac{\partial T}{\partial x}\]
Re-solve the horizontal convection problem (P10.27) with this new condition, assuming that the surface tension varies linearly with the temperature.
\[\frac{\partial \gamma}{\partial T}=-\beta_{\gamma}\]
Problem 10.27
We would like to look at a case of coupled transport: natural convection between horizontal walls. The situation is shown in Figure P10.27. We assume that the length of the device is much larger than its height, i.e., vy≈0 and vx=f(y).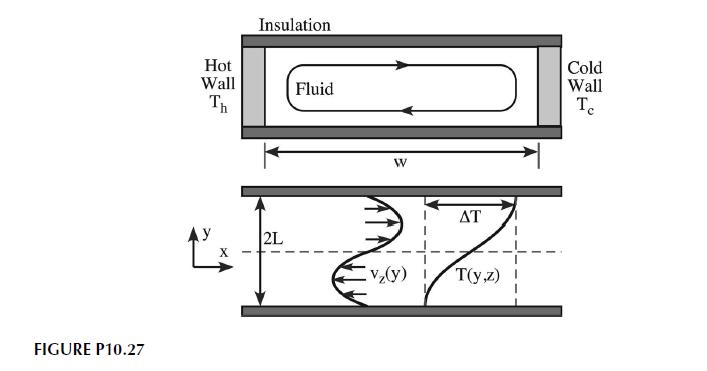
Step by Step Answer:







