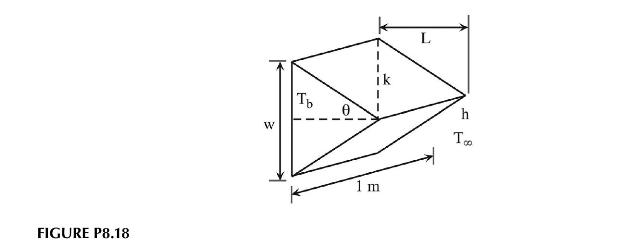
Triangular shaped fins offer high heat transfer capability and low weight when compared to other geometries. Consider the triangular fin whose base is held a constant temperature of \(T_{b}\). Since the cross-sectional area of the fin is zero at its tip, the location poses a problem. Resolve it by changing the coordinate system to make the fin tip at \(x=0\) and its base at \(x=L\). The free-stream temperature is \(T_{\infty}\). For the fin dimensions shown in Figure P8.18, show that the temperature profile is given by:

\[\theta=\frac{T-T_{\infty}}{T_{b}-T_{\infty}}=\frac{I_{o}(2 \alpha \sqrt{x})}{I_{o}(2 \alpha)} \quad \alpha^{2}=\frac{h L}{k \sin \theta}\]

Transcribed Image Text:

FIGURE P8.18 W Tb 1 m L T h