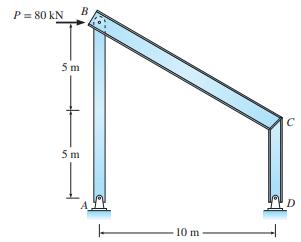
For the steel frame in Figure P8.41, compute the horizontal displacement of joint \(B\). For member \(B C D\), \(A=6000 \mathrm{~mm}^{2}\) and \(I=600 \times 10^{6} \mathrm{~mm}^{4}\). For member \(A B\), \(A=3000 \mathrm{~mm}^{2} . E=200 \mathrm{GPa}\) for all members.

This note applies to Problems P8.42 to P8.44.

Because reinforced concrete beams crack due to tensile stresses created by moment and shear, initial elastic deflections are based on an empirical equation for moment of inertia established from experimental studies of full-size beams (provided in the ACI Code). This equation produces an effective moment of inertia \(I_{e}\) that varies from about 0.35 to 0.5 of the moment of inertia \(I_{G}\) based on the gross area of the cross section. The additional deflection due to creep and shrinkage that occurs over time, which can exceed the initial deflection, is not considered.

Transcribed Image Text:

P = 80 KN B 5m 5m 10 m C D