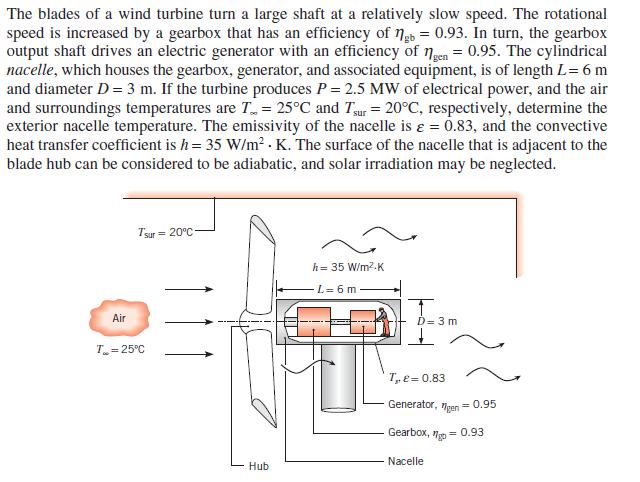
Consider the nacelle of the wind turbine in Example 1.3. The nacelle is formed of a thermoplastic composite material of thickness \(L=20 \mathrm{~mm}\). The thermal conductivity, density, and specific heat of the nacelle material are \(k=1.5 \mathrm{~W} / \mathrm{m} \cdot \mathrm{K}, ho=1250 \mathrm{~kg} / \mathrm{m}^{3}\), and \(c=1500 \mathrm{~J} / \mathrm{kg} \cdot \mathrm{K}\). In reality, the heat transfer coefficient at the exterior nacelle surface is not constant, but varies as the blades rotate about the nacelle leading to a sinusoidal variation in the heat transfer coefficient, \(h(t)=\bar{h}+\Delta h \sin (\omega t)\). The values of \(\bar{h}\) and \(\Delta h\) are \(35 \mathrm{~W} / \mathrm{m}^{2} \cdot \mathrm{K}\) and \(15 \mathrm{~W} / \mathrm{m}^{2} \cdot \mathrm{K}\), respectively. The frequency \(\omega\) may be determined from the number of blades (3) and the rotational speed of the blades (17 rpm).

At time \(t=0\), the temperature distribution through the wall is given by \(T(x, t=0)=A x+B\), where \(x=0\) corresponds to the interior nacelle surface, \(A=\) \(-3460^{\circ} \mathrm{C} / \mathrm{m}\), and \(B=212^{\circ} \mathrm{C}\). Using this initial condition, determine the temperature distribution within the wall \(T(x, t)\) over the time period \(40 \leq t \leq 50 \mathrm{~s}\). Assume that the inner surface of the nacelle is maintained at its initial value of \(212^{\circ} \mathrm{C}\). Use a grid spacing of \(\Delta x=2 \mathrm{~mm}\) and a time step of \(\Delta t=0.05 \mathrm{~s}\). Plot the exterior nacelle surface temperature, the exterior surface convection heat flux, and the exterior surface radiation heat flux over the time period \(40 \leq t \leq 50 \mathrm{~s}\). Explain the physical basis for the phenomena you observe from your solution. 

Data From Example 1.3:-

Transcribed Image Text:

The blades of a wind turbine turn a large shaft at a relatively slow speed. The rotational speed is increased by a gearbox that has an efficiency of 1gb = 0.93. In turn, the gearbox output shaft drives an electric generator with an efficiency of gen = 0.95. The cylindrical nacelle, which houses the gearbox, generator, and associated equipment, is of length L = 6 m and diameter D = 3 m. If the turbine produces P = 2.5 MW of electrical power, and the air and surroundings temperatures are T = 25C and Tsur = 20C, respectively, determine the exterior nacelle temperature. The emissivity of the nacelle is = 0.83, and the convective heat transfer coefficient is h = 35 W/m. K. The surface of the nacelle that is adjacent to the blade hub can be considered to be adiabatic, and solar irradiation may be neglected. Air = Tsur 20C T=25C h=35 W/mK L=6m D= 3 m Hub T = 0.83 Generator, gen = 0.95 Gearbox, 17gp = 0.93 Nacelle