Question:
The spectral transmissivity of a 1-mm-thick layer of liquid water can be approximated as follows:
\[\begin{array}{ll}\tau_{\lambda 1}=0.99 & 0 \leq \lambda \leq 1.2 \mu \mathrm{m} \\\tau_{\lambda 2}=0.54 & 1.2 \mu \mathrm{m}
(a) Liquid water can exist only below its critical temperature, \(T_{c}=647.3 \mathrm{~K}\). Determine the maximum possible total transmissivity of a 1 -mm-thick layer of liquid water when the water is housed in an opaque container and boiling does not occur. Assume the irradiation is that of a blackbody.
(b) Determine the transmissivity of a 1-mm-thick layer of liquid water associated with melting the platinum wire used in Nukiyama's boiling experiment, as described in Section 10.3.1.
(c) Determine the total transmissivity of a 1-mm-thick layer of liquid water exposed to solar irradiation. Assume the sun emits as a blackbody at \(T_{s}=\) \(5800 \mathrm{~K}\).
Data From Section 10.3.1:-
Transcribed Image Text:
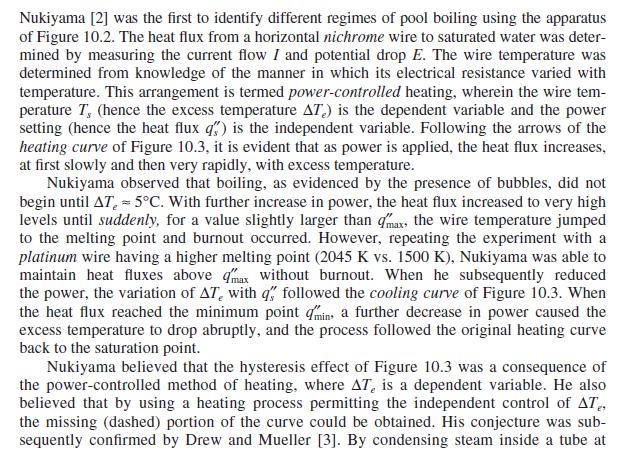
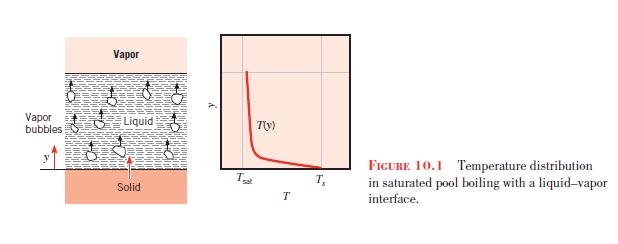
Nukiyama [2] was the first to identify different regimes of pool boiling using the apparatus of Figure 10.2. The heat flux from a horizontal nichrome wire to saturated water was deter- mined by measuring the current flow I and potential drop E. The wire temperature was determined from knowledge of the manner in which its electrical resistance varied with temperature. This arrangement is termed power-controlled heating, wherein the wire tem- perature T, (hence the excess temperature AT) is the dependent variable and the power setting (hence the heat flux ") is the independent variable. Following the arrows of the heating curve of Figure 10.3, it is evident that as power is applied, the heat flux increases, at first slowly and then very rapidly, with excess temperature. Nukiyama observed that boiling, as evidenced by the presence of bubbles, did not begin until AT=5C. With further increase in power, the heat flux increased to very high levels until suddenly, for a value slightly larger than maxs the wire temperature jumped to the melting point and burnout occurred. However, repeating the experiment with a platinum wire having a higher melting point (2045 K vs. 1500 K), Nukiyama was able to maintain heat fluxes above max without burnout. When he subsequently reduced the power, the variation of AT, with q" followed the cooling curve of Figure 10.3. When the heat flux reached the minimum point dmins a further decrease in power caused the excess temperature to drop abruptly, and the process followed the original heating curve back to the saturation point. Nukiyama believed that the hysteresis effect of Figure 10.3 was a consequence of the power-controlled method of heating, where AT, is a dependent variable. He also believed that by using a heating process permitting the independent control of AT, the missing (dashed) portion of the curve could be obtained. His conjecture was sub- sequently confirmed by Drew and Mueller [3]. By condensing steam inside a tube at









