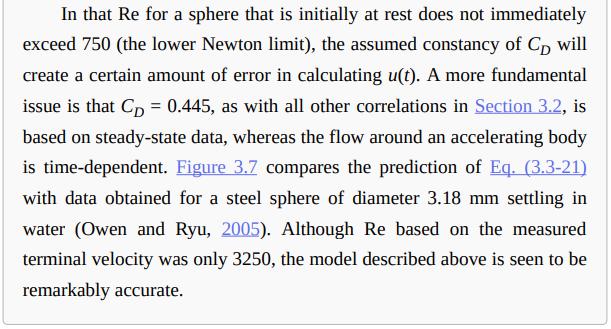
An object moving through a static fluid sets part of the fluid in motion, and if it accelerates, the neighboring fluid must accelerate as well. The acceleration of the fluid tends to slow the acceleration of the object. As discussed in Example 3.3-3, this may be accounted for by assigning to the object an effective mass that exceeds its actual mass. The increment, or added mass, is calculated most easily for potential flow, and the objective is to evaluate it for a solid sphere of radius R. Assume that the sphere moves at a velocity U(t) in the z direction and that the inviscid fluid of density ρ is otherwise at rest.

(a) Supposing that U(t) is known, find the velocity potential ϕ(r, θ, t). (The general solution for ϕ is the same as in Problem 9.1, but now the boundary conditions in r must correspond to a sphere that is moving and distant fluid that is stationary.)

(b) Evaluate

by applying Eq. (9.2-14) at the sphere surface.

(c) Show that the z component of the fluid-dynamic force on the sphere is

The negative sign confirms that displacing the surrounding fluid slows the sphere, and the coefficient of dU⁄dt shows that the added mass is (2π/3)R³ρ, or half the mass of the displaced fluid. The fact that there is not also a contribution to F_z that involves U (as opposed to dU⁄dt) reflects the absence of drag in steady potential flow.

Transcribed Image Text:

P(R, 0, 1)