The objective is to estimate the drag on a cylinder from the velocity reduction measured in its
Question:
The objective is to estimate the drag on a cylinder from the velocity reduction measured in its wake. The cylinder of diameter d and length L (≫ d) is perpendicular to the approaching flow. Figure P11.5 shows a control volume that includes half the system of interest. It extends from a position upstream from the cylinder (S1, where vx = U) to one many diameters downstream (S2, where vx is measured). To ensure that vx = U everywhere on S3, let H → ∞. Except for the part that coincides with the top of the cylinder, S4 is a symmetry plane.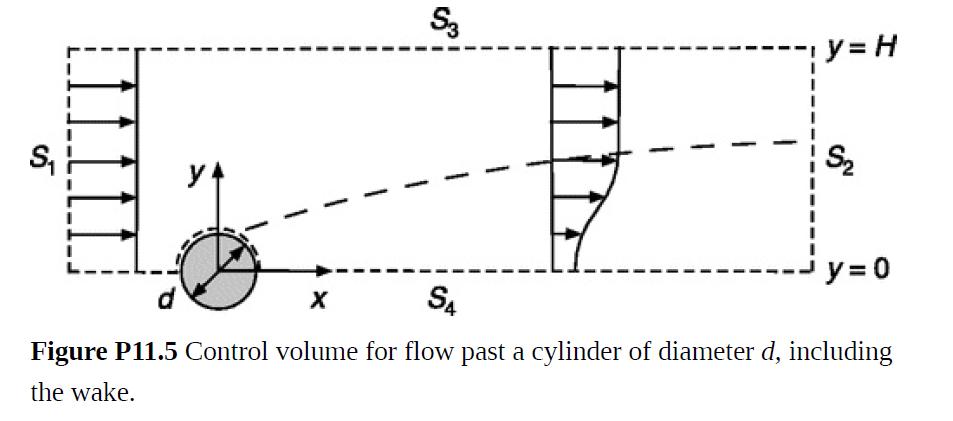
(a) Show that the outward flow rate through S3 is given by an expression like Eq. (P11.4-1).
(b) Show that the drag can be calculated much as in Eq. (P11.4-2).
(c) Of particular interest is the velocity reduction at S2, Λ(x, y) = U − vx(x, y). If Λ/U ≪ 1, show that the drag coefficient for the full cylinder is given by
(d) Townsend (1949) mounted a wire with d = 0.159 cm in a wind tunnel. For U = 12.80 m/s, corresponding to Re = 1360, he measured wake velocities at positions within the range 500 ≤ x⁄d ≤ 950. The data were represented fairly well (although not exactly) by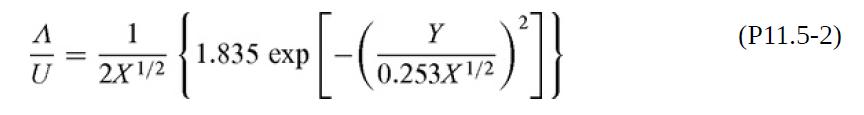
where Y = y⁄d, X = (x − x0)/d, and x0/d = 90. Evaluate CD from this information. [Equation (P11.4-5) will be helpful.] 
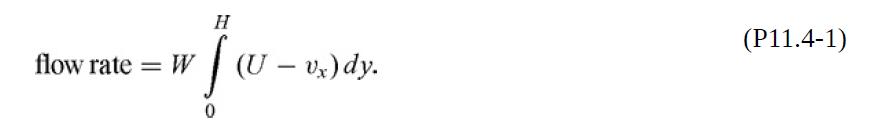

Step by Step Answer:

Introduction To Chemical Engineering Fluid Mechanics
ISBN: 9781107123779
1st Edition
Authors: William M. Deen





