The drag on an object can be inferred from the velocity reduction in its wake. Figure P11.4
Question:
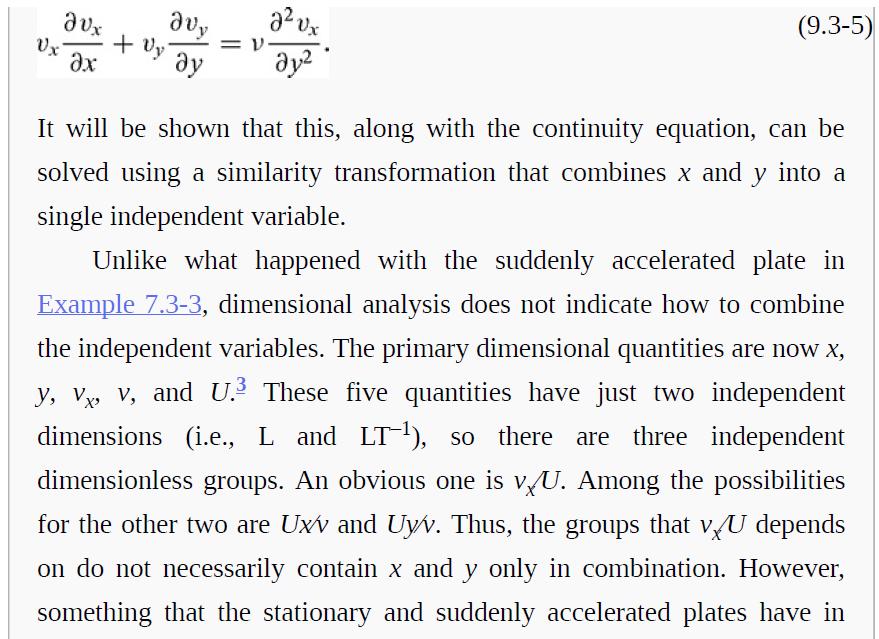
The drag on an object can be inferred from the velocity reduction in its wake. Figure P11.4 depicts the boundary layer and wake on one side of a flat plate. The surfaces S1 through S4 enclose a control volume that extends from y = 0 to y = H and from a position upstream from the leading edge (where vx = U) to one downstream from the trailing edge. To ensure that vx = U everywhere on S3, let H → ∞. The plate length is L and its width is W.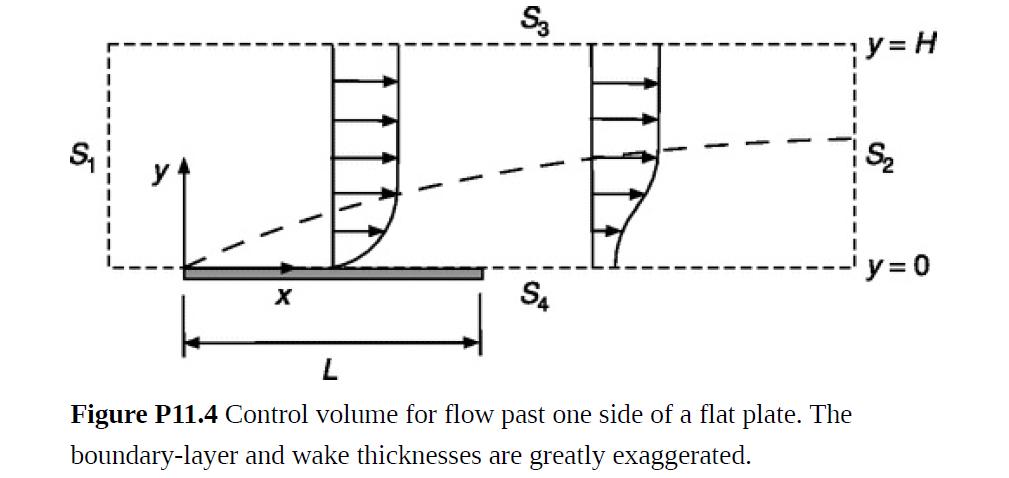
(a) Show that the outward volume flow rate through S3 is related to U and the velocity vx evaluated on S2 as
(b) Show that the drag on one side of the plate is
where vx again is evaluated on S2.
(c) For x⁄L > 3, it has been found that the velocity reduction, Λ(x, y) = U − vx(x, y), is represented accurately by (Schlichting, 1968, p. 169)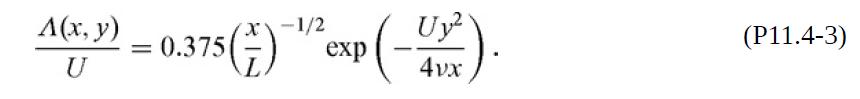
For x large enough that Λ/U ≪ 1, vx≅U and the result in part (b) can be simplified to
Use this to calculate Cf and compare your result with that in Example 9.3-1. A definite integral that is helpful here is
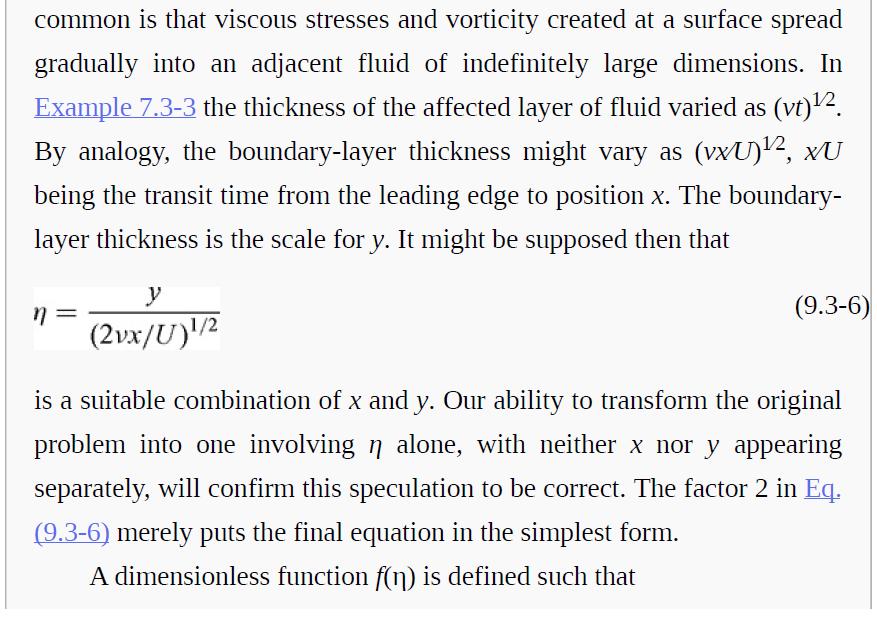
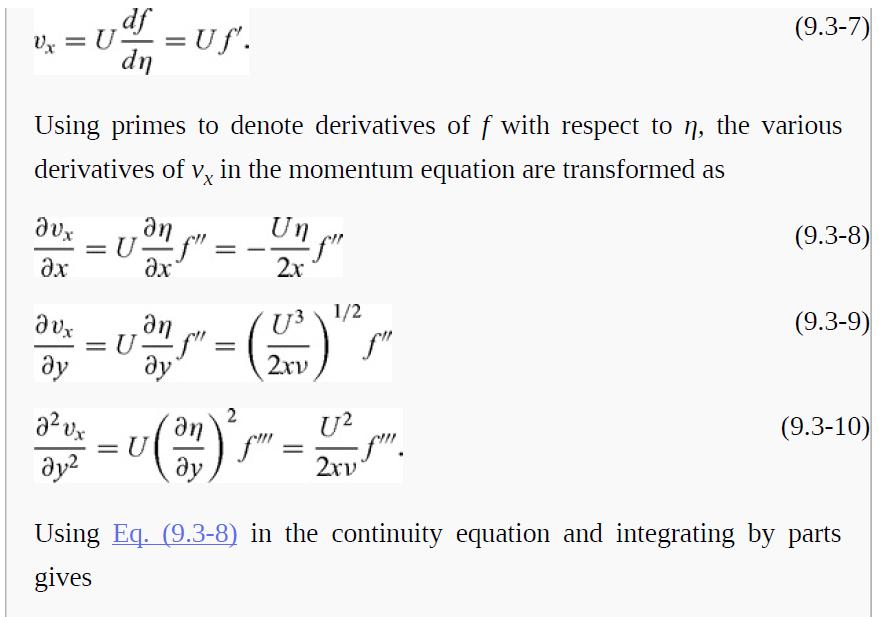
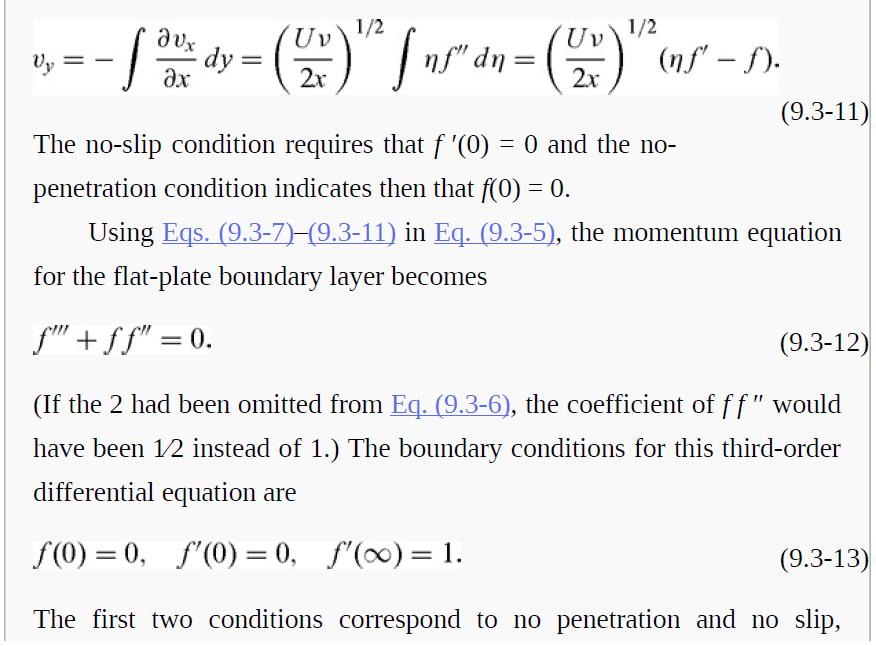


Step by Step Answer:

Introduction To Chemical Engineering Fluid Mechanics
ISBN: 9781107123779
1st Edition
Authors: William M. Deen





