a. In Problem 42, the capacity of the college is 3,000 students. If the university plans to
Question:
a. In Problem 42, the capacity of the college is 3,000 students. If the university plans to increase the capacity of the college to 3,500, should the college accept this expansion? Explain.
b. Analyze the number of students admitted when the capacity is 3,000 and when it is increased to 3,500.
Data From Problem 42
The college of business of a large university offers six major programs and set the tuition as tabulated.
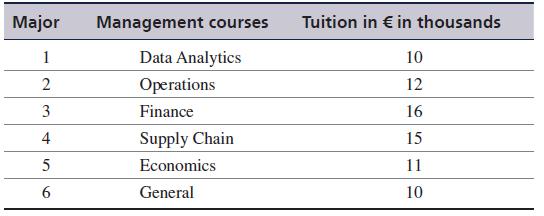
The college plans to admit a total of 3,000 students in all the six majors with at least 200 students in each major. Each major should admit no more than 20% students admitted to the college. Majors 3 through 6 are using common resources. To avoid shortage of resources, students admitted in Finance should be at most 25% of the total students admitted in Supply Chain, Economics, and General together. Each major should have students, otherwise the university will close the major program. The university wants to determine the number of students it should admit in each major to maximize its tuition revenue.
a. Formulate a linear programming model.
b. Using computer, solve the model.
Step by Step Answer:
a A 20000 ft 2 increase in store size to 160000 ft 2 would ...View the full answer
