Question: An engineer is measuring a quantity q. It is assumed that there is a random error in each measurement, so the engineer will take n

An engineer is measuring a quantity q. It is assumed that there is a random error in each measurement, so the engineer will take n measurements and reports the average of the measurements as the estimated value of q. Specifically, if Y_i is the value that is obtained in the i'th measurement, we assume that Y_i = q +X_i, where X_i is the error in the ith measurement. We assume that X_i's are i.i.d. with EX_i = 0 and Var(X_i) = 4 units. The engineer reports the average of measurements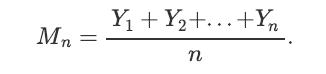 Mn Y+Y+...+Yn n

How many measurements does the engineer need to make until he is 95% sure that the final error is less than 0.1 units? In other words, what should the value of n be such that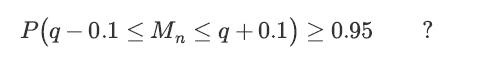 P(q-0.1 M q +0.1) 0.95 ?

Mn Y+Y+...+Yn n

Step by Step Solution

★★★★★

3.43 Rating (156 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

To find the number of measurements n needed such that the engineer i... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Introduction To Probability Statistics Questions!

An engineer is measuring a quantity . It is assumed that there is a random error in each measurement, so the engineer will take n measurements and report the average of the measurements as the...

The Crazy Eddie fraud may appear smaller and gentler than the massive billion-dollar frauds exposed in recent times, such as Bernie Madoffs Ponzi scheme, frauds in the subprime mortgage market, the...

Planning is one of the most important management functions in any business. A front office managers first step in planning should involve determine the departments goals. Planning also includes...

\fThis is an electronic version of the print textbook. Due to electronic rights restrictions, some third party content may be suppressed. Editorial review has deemed that any suppressed content does...

Experiment 3 Acceleration Due To Gravity Questions How do you compute an object's velocity from its position? How do you measure the acceleration of a mass as it falls under the influence of gravity?...

413-432_CH18_Nachmias.qxd 1/17/07 3:47 PM Page 413 Chapter Index Construction and Scaling Methods INDEX CONSTRUCTION The Purpose of the Index The Sources of the Data The Base of Comparison Methods of...

Please I need help with the questions for Calculations (Part ), Calculations (Part 2), the graph, and questions 1-2 on the last page. THE DATA TO DO THE CALCULATIONS ARE PROVIDED IN THE TABLES. THATS...

I need help with this lab. Please answer questions for pages 4-7. Freefall Purpose: We will measure the value of 'little g', or the acceleration due to gravity on the surface of Earth. The particular...

Jupiter Notebook We have covered some of the limitations of single layer neural networks in class, but they are still powerful learning systems that provide a good way to begin learning about how to...

ASAP!!! I need help with a lab report and need to include my week 1 experiment data and week 2 experiment data into an essay. The instructions for the lab report is provided as well as my data for...

Floress Catering purchased equipment that cost $2,500 but it was recorded as $520. Which of the following statement(s) are correct? a. This is a transposition error. b. This is a slide error. c. This...

If you had a 15,000 pesos budget for 30 days, considering you also pay utilities, how would you budget it with the following expenses: a. consumption (food) b. clothing c. health d. savings Explain...

Is the following equivalence relation true of false? Justify your answer. ( r 1 + r 2 ) * = ( r 1 * r 2 * ) *

What OCEAN personality traits, intelligence components, or EQ components do you think helped political leaders successfully navigate the run-up, lockdown, and economic restart phases of the...

Damages at a factory manufacturing chairs are categorized according to the material wasted. plastic 75 iron 31 cloth 22 spares 8 Draw a Pareto chart.

Refer to Example 12 of motors for miniaturized capsules, but instead suppose that 20 motors are available and that 4 will not operate satisfactorily, when placed in a capsule. If the scientist wishes...

Refer to Example 31 concerning spam but now suppose that among the 5000 messages, the 1750 spam messages have 1570 that contain the words on a new list and that the 3250 normal messages have 300 that...

help please 1. When the partnership goes out of business and the normal operations are discontinued, the accounts should be adjusted and dosed ercept a. the asset accounts. b. the liability accounts....

Question 30 (2 points) A company wrote a cheque for $329 to pay for supplies but it was incorrectly recorded on the books as $239. The bank properly processed the cheque as $329. On a bank...

Mr. Kelly owns stock in VP and in BL, both of which are S corporations. This year, he had the following income and loss items: Salary Business income from VP Business loss from BL $ 62,590 19,300...