Let W(t) and U(t) be two independent standard Brownian motions. Let 1 1. Define

Let W(t) and U(t) be two independent standard Brownian motions. Let −1 ≤ ρ ≤ 1. Define the random process X(t) as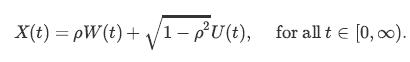

a. Show that X(t) is a standard Brownian motion.

b. Find the covariance and correlation coefficient of X(t) and W(t). That is, find Cov(X(t),W(t)) and ρ(X(t),W(t)).

Transcribed Image Text:

X(t) = pW (t) + √√1 - p²U(t), for all t = [0, ∞).