Supersymmetry. Consider the two operators for some function W (x) . These may be multiplied in either
Question:
Supersymmetry. Consider the two operators
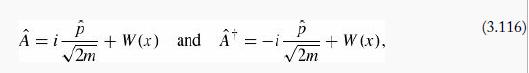
for some function W (x) . These may be multiplied in either order to construct two Hamiltonians:
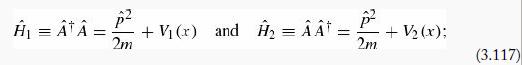
V1 and V2 are called supersymmetric partner potentials. The energies and eigenstates of Ĥ1 and Ĥ2 are related in interesting ways.
(a) Find the potentials V1(x) and V2(x), in terms of the superpotential, w(x).
(b) Show that if Ψn(1) is an eigenstate of Ĥ1 with eigenvalue En(1), then ÂΨn(1) is an eigenstate of Ĥ2 with the same eigenvalue. Similarly, show that if Ψn(2) (x) is an eigenstate of Ĥ2 with eigenvalue En(2), then ÂΨn(2)is an eigenstate of Ĥ1 with the same eigenvalue. The two Hamiltonians therefore have essentially identical spectra.
(c) One ordinarily chooses W(x) such that the ground state of Ĥ1 satisfies

and hence E0(1) = 0. Use this to find the superpotential W(x), in terms of the ground state wave function, Ψ0(1) (x). (The fact that  annihilates
Ψ0(1) means that Ĥ2 actually has one less eigenstate than Ĥ1, and is missing the eigenvalue E0(1).)
(d) Consider the Dirac delta function well,

(the constant term, ma2/2ћ2, is included so that E0(1) = 0). It has a single bound state (Equation 2.132)
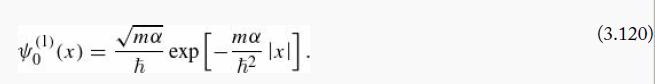
Use the results of parts (a) and (c), and Problem 2.23(b), to determine the superpotential W(x) and the partner potential V2(x). This partner potential is one that you will likely recognize, and while it has no bound states, the supersymmetry between these two systems explains the fact that their reflection and transmission coefficients are identical (see the last paragraph of Section 2.5.2).
Step by Step Answer:

Introduction To Quantum Mechanics
ISBN: 9781107189638
3rd Edition
Authors: David J. Griffiths, Darrell F. Schroeter





