5.3 Consider a problem with four control factors and three noise factors. Each factor has two levels.

(a) In crossed arrays, howmany runs are needed to avoid two-factor interactions to be confounded with main effects or another two-factor interaction (to get a design of resolution V or higher)?

(b) Howmany runs are needed in a combined array under the same requirement?

(c) How many runs are needed in a combined array to avoid the situation where control-by-noise interactions are confounded with main effects or other two-factor interactions?

(d) In crossed arrays, how does the confounding pattern look if the inner array is of the type 24−1 and the outer array 23? [Hint: write all runs in a common array to simplify the analysis.]

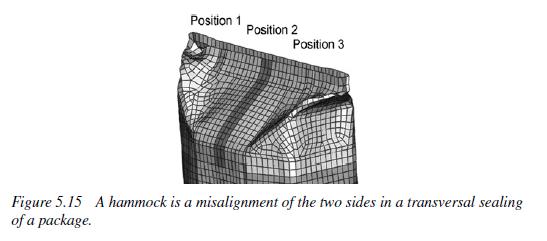
(e) Compare the crossed arrays in

(d) with an ordinary half fractional combined array, 27−1, in the same way as in Table 5.15.

(f) In (d), the inner array is fractional but the outer one is not. Would there be any advantages or drawbacks to fractionate the outer array but not the inner array?

Transcribed Image Text:

Position 1 Position 2 Position 3 Figure 5.15 A hammock is a misalignment of the two sides in a transversal sealing of a package.