Let \(B_{1}, \ldots, B_{n}\) be a set of independent and identically distributed random variables from a \(\operatorname{Bernoulli}(\theta)\) distribution. Suppose we are interested in estimating the variance \(g(\theta)=\theta(1-\theta)\) with the estimator \(g\left(\bar{B}_{n}ight)=\) \(\bar{B}_{n}\left(1-\bar{B}_{n}ight)\).

a. Find the bias and variance of \(g\left(\bar{X}_{n}ight)\) as an estimator of \(g(\theta)\) directly.

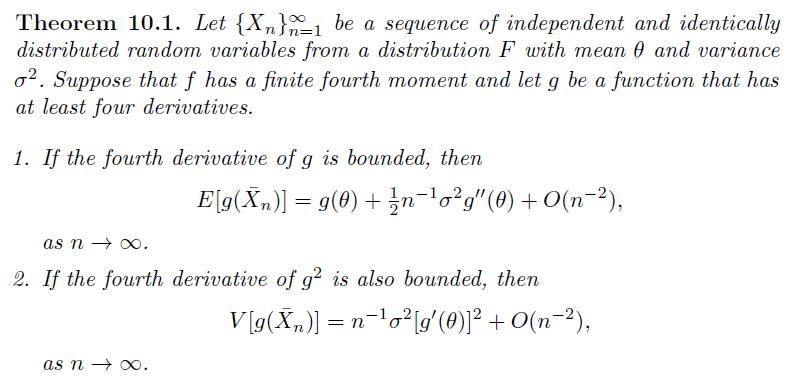
b. Find asymptotic expressions for the bias and variance of \(g\left(\bar{X}_{n}ight)\) as an estimator of \(g(\theta)\) using Theorem 10.1. Compare this result to the exact expressions derived above.

Transcribed Image Text:

Theorem 10.1. Let {X}1 be a sequence of independent and identically distributed random variables from a distribution F with mean 0 and variance 02. Suppose that f has a finite fourth moment and let g be a function that has at least four derivatives. 1. If the fourth derivative of g is bounded, then as n. -1 E[g(Xn)] = g(0) + nog (0) + O(n), 2. If the fourth derivative of g is also bounded, then as n. V[g(Xn)] = ng'(0)] + O(n),