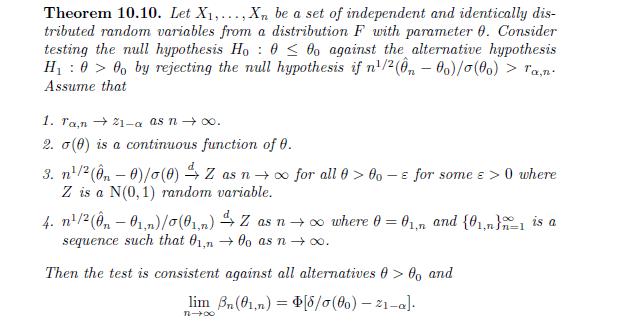
Let \(X_{1}, \ldots, X_{n}\) be a set of independent and identically distributed random variables from a distribution \(F\) with parameter \(\theta\). Consider testing the null hypothesis \(H_{0}: \theta \geq \theta_{0}\) against the alternative hypothesis \(H_{1}: \theta

a. Prove that the test is consistent against all alternatives \(\theta

b. Develop an expression similar to that given in Theorem 10.10 for the asymptotic power of this test for a sequence of alternatives given by \(\theta_{1, n}=\theta_{0}-n^{-1 / 2} \delta\) where \(\delta>0\) is a constant. State any additional assumptions that must be made in order for this result to be true.

Transcribed Image Text:

Theorem 10.10. Let X,..., Xn be a set of independent and identically dis- tributed random variables from a distribution F with parameter 6. Consider testing the null hypothesis Ho00o against the alternative hypothesis H:00 by rejecting the null hypothesis if n/2(n-00)/(00) > Tan Assume that 1. Tan 21-a as n . 2. (0) is a continuous function of 0. 3. n/2 (0-0)/(0) Z as n for all >00 - for some => 0 where Z is a N(0, 1) random variable. 4. n/2(n-01,n)/(01,n) Z as n where 0 = 01, and {01,n}=1 is a sequence such that 01,00 as n . Then the test is consistent against all alternatives > 0 and lim Br(n,n) = [6/a(%) z1_a]. 8