a. Show that the mean-squared forecast error (Eleft[left(hat{y}_{T+1}-y_{T+1} ight)^{2} mid I_{T} ight]) for a forecast (hat{y}_{T+1}), that

a. Show that the mean-squared forecast error \(E\left[\left(\hat{y}_{T+1}-y_{T+1}\right)^{2} \mid I_{T}\right]\) for a forecast \(\hat{y}_{T+1}\), that depends only on past information \(I_{T}\), can be written as

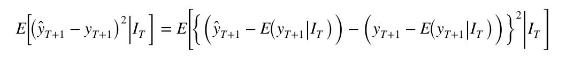
b. Show that \(E\left[\left(\hat{y}_{T+1}-y_{T+1}\right)^{2} \mid I_{T}\right]\) is minimized by choosing \(\hat{y}_{T+1}=E\left(y_{T+1} \mid I_{T}\right)\).

Transcribed Image Text:

+1, - [87-ru)] = {(xen (r)) - (you-Eltz))}*|4]