Consider the wage model in Example 10.5 and the 428 observations on married women who participate in the labor force. Use only MOTHEREDUC as an instrument in this exercise.

a. Estimate the first-stage equation by OLS and obtain the fitted values

b. Use OLS to estimate the second-stage equation

c. Obtain the least squares residuals, \(\hat{e}\), from the estimation in part (b). Calculate \(\sum \hat{e}_{i}\). Explain why the value you obtain is theoretically correct.

d. Using the coefficient estimates from part (b), calculate the residuals

Calculate \(\sum \hat{e}_{I V}\). Explain why the value you obtain is theoretically correct.

e. Calculate \(\sum \hat{e}_{i}^{2} /(N-4)\) and \(\sum \hat{e}_{I V}^{2} /(N-4)\). Which of these is the correct estimator of the error variance, \(\sigma^{2}\) ?

f. Estimate the regression \(\widehat{E D U C}=a_{1}+a_{2} E X P E R+a_{3} E X P E R^{2}+\) error and obtain the sum of squared residuals. Use equation (10.25) and one of the values from part (e) to obtain \(\widehat{\operatorname{var}}\left(\hat{\beta}_{4}\right)\).
g. Using software for IV/2SLS estimate the wage model \(\ln (W A G E)=\beta_{1}+\beta_{2} E X P E R+\beta_{3} E X P E R^{2}+\) \(\beta_{4} E D U C+e\) using the instrumental variable MOTHEREDUC. How do the estimates compare to those in part (b)? Does the reported standard error se \(\left(\hat{\beta}_{4}\right)\) agree with the calculated variance in part (f)?

Data From Example 10.5:-

In addition to education a worker's experience is also important in determining their wage. Because additional years of experience have a declining marginal effect on wage use the quadratic model 

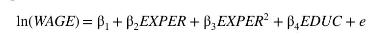
where EXPER is years of experience. This is the same specification. We assume that EXPER is an exogenous variable that is uncorrelated with the worker's ability and therefore uncorrelated with the random error \(e\). Two instrumental variables for years of education, \(E D U C\), are mother's and father's years of education, MOTHEREDUC and FATHEREDUC, introduced in the previous examples. The first-stage equation is  

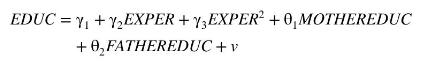
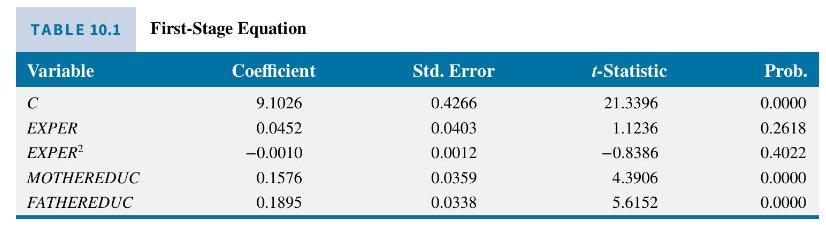
Using the 428 observations in the data file mroz the estimated first-stage equation is reported in Table 10.1. The IV/2SLS estimates, with correctly computed standard errors, are

The estimated return to education is approximately \(6.1 \%\), and the estimated coefficient is statistically significant with a \(t=1.96\).

Transcribed Image Text:

EDUC=+EXPER +EXPER + , MOTHEREDUC