Households plan consumption expenditures and saving with consideration of their long-run income. We wish to estimate SAVING \(=\beta_{1}+\beta_{2}\) LRINCOME \(+e\), where LRINCOME is long-run income.

a. Long-run income is difficult to define and measure. Using data on 50 households' annual savings (SAVINGS, \\($1000\) units) and annual income (INCOME, \\($1000\) units), we estimate a savings equation by OLS to obtain

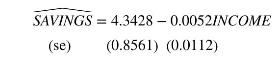
Why might we expect the OLS estimator of the marginal propensity to save to be biased and inconsistent? What is the likely direction of the bias?

b. Suppose that in addition to current income we know average household income over the past 10 years (AVGINC, \(\$ 1000\) units). Why might this be a suitable instrumental variable?

c. The estimated first-stage regression is

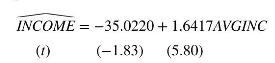
Does AVGINC qualify as a strong instrument? Explain.

d. Let the residuals from part (c) be \(\hat{v}\). Adding this variable to the savings equation and estimating the result by OLS gives

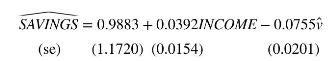
Based on this result should we rely on the OLS estimates of the savings equation?

e. Using the fitted values from part (c) in place of INCOME and applying OLS, we obtain

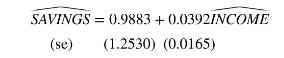
Compare these coefficient estimates to those in part (a). Are these estimates more in line with your prior expectations than those in (a), or not?

f. Are the OLS standard errors in part (e) correct or not? Explain.

g. Using IV/2SLS software, with instrument \(A V G I N C\), we obtain the estimates

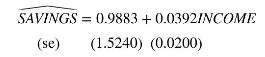
Construct a 95\% interval estimate of the effect of INCOME on SAVINGS. Compare and contrast it to the \(95 \%\) interval estimate based on the results in part (a).
h. In parts (d), (e), and (g), the estimated coefficient of INCOME is 0.0392 . Is this an accident? Explain.
i. Explain how to test whether \(A V G I N C\) is a valid instrument, and uncorrelated with the regression error.

Transcribed Image Text:

SAVINGS 4.3428 -0.0052/NCOME = (se) (0.8561) (0.0112)