The process of adding rational functions (ratios of polynomials) by placing them over a common denominator is
Question:
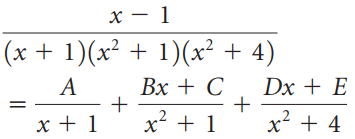
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Question Posted:





