Real and nominal exchange rates and inflation Using the definition of the real exchange rate (and Propositions 7 and 8 in Appendix 2 at the end of the book), you can show that

\[
\frac{\left(\varepsilon_{t}-\varepsilon_{t-1}ight)}{\varepsilon_{t-1}}=\frac{\left(E_{t}-E_{t-1}ight)}{E_{t-1}}+\pi_{t}-\pi_{t}^{*}
\]

In words, the percentage real appreciation equals the percentage nominal appreciation plus the difference between domestic and foreign inflation.

a. If domestic inflation is higher than foreign inflation, and the domestic country has a fixed exchange rate, what happens to the real exchange rate over time? Assume that the Marshall-Lerner condition holds. What happens to the trade balance over time? Explain in words.

b. Suppose the real exchange rate is currently at the level required for net exports (or the current account) to equal zero. In this case, if domestic inflation is higher than foreign inflation, what must happen over time to maintain a trade balance of zero?

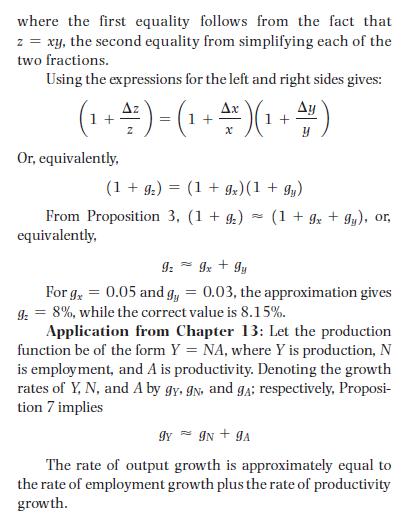
Proposition 7

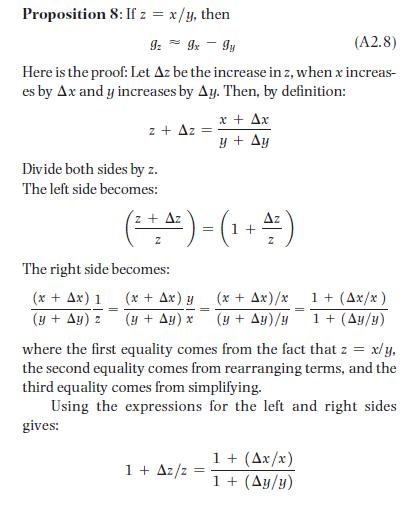
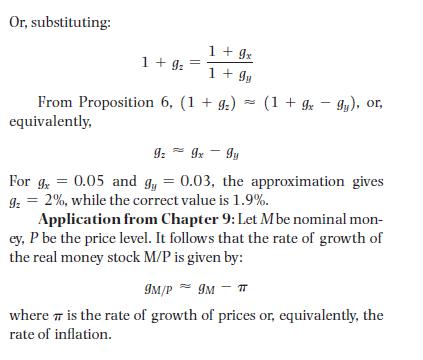
Proposition 8

Transcribed Image Text:

Proposition 7: If z = xy then: 9= = 9x + gy (A2.7) Here is the proof: Let Az be the increase in zwhen x increases by Ax and y increases by Ay. Then, by definition: z+Az = (x + Ax) (y + Ay) Divide both sides by z. The left side becomes: (z+ Az) Z The right-hand side becomes (x + Ax) (y + Ay) Z Az (1 + 4/) (x + Ax) (y + Ay) y x Ax Ay - ( 1 + A ) ( 1 + ) = x y