A confidence interval for the regression coefficient 1 is expressed as where The critical t score

A confidence interval for the regression coefficient β₁ is expressed as

where

The critical t score is found using n - (k + 1) degrees of freedom, where k, n, and s_b1 are described in Exercise 17. Using the sample data from Example 1, n = 153 and k = 2, so df = 150 and the critical t scores are ± 1.976 for a 95% confidence level. Use the sample data for Example 1, the Statdisk display in Example 1, and the StatCrunch display in Exercise 17 to construct 95% confidence interval estimates of β₁ (the coefficient for the variable representing height) and β₂ (the coefficient for the variable representing waist circumference). Does either confidence interval include 0, suggesting that the variable be eliminated from the regression equation?

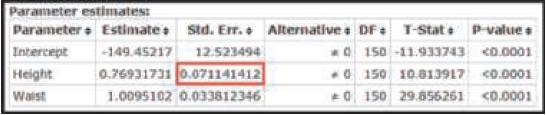
Exercise 17:

If the coefficient β₁ has a nonzero value, then it is helpful in predicting the value of the response variable. If β1 = 0, it is not helpful in predicting the value of the response variable and can be eliminated from the regression equation. To test the claim that β₁ = 0 use the test statistic t = (b1 - 0)/s_b1. Critical values or P-values can be found using the t distribution with n - (k + 1) degrees of freedom, where k is the number of predictor (x) variables and n is the number of observations in the sample. The standard error s_b1 is often provided by software. For example, see the accompanying StatCrunch display for Example 1, which shows that s_b1 = 0.071141412 (found in the column with the heading of "Std. Err." and the row corresponding to the first predictor variable of height). Use the sample data in Data Set 1 "Body Data" and the StatCrunch display to test the claim that β₁ = 0. Also test the claim that β₂ = 0. What do the results imply about the regression equation?