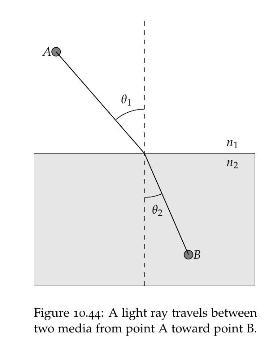
A light ray travels from point \(\mathrm{A}\) in a medium with index of refraction \(n_{1}\) toward point \(B\) in a medium with index of refraction \(n_{2}\). Assume that the rays travel in straight lines and bend at a point at the interface as shown in Figure 10. 44. The time functional is

\[T[y]=\int \frac{d s}{v}\]

a. Write the time functional in terms of the travel path \(y(x)\).

b. Apply Fermat's Principle of least time to write the Euler Equation for this functional.

c. Solve the equation in part \(\mathrm{b}\). and show that \(n \sin \theta\) is a constant.

d. Let the point at which the light is incident to the interface be at \((x, 0)\). Write an expression for the total time to travel from point \(A\) at \(\left(x_{1}, y_{1}\right)\) to point \(B\) at \(\left(x_{2}, y_{2}\right)\) in terms of the indices of refraction and the coordinates \(x, x_{1}, x_{2}\), and \(y_{2}\).

e. Treating the time as a function of \(x\), minimize this function as a function of one variable and derive Snell's law of refraction.

OB 111 112 Figure 10.44: A light ray travels between two media from point A toward point B.