Consider a floor on composition, where the composition is defined as 

Here, L_i is reset at time T_i and α_i+1 is the accrual factor over the time interval (T_i,T_i+1]. The payment of this floor at maturity T_n is

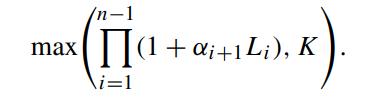
Assuming that the discounted bond price process follows the same process as in Problem 8.21, show that the time-0 value of this floor on composition is given by (Henrard, 2005)

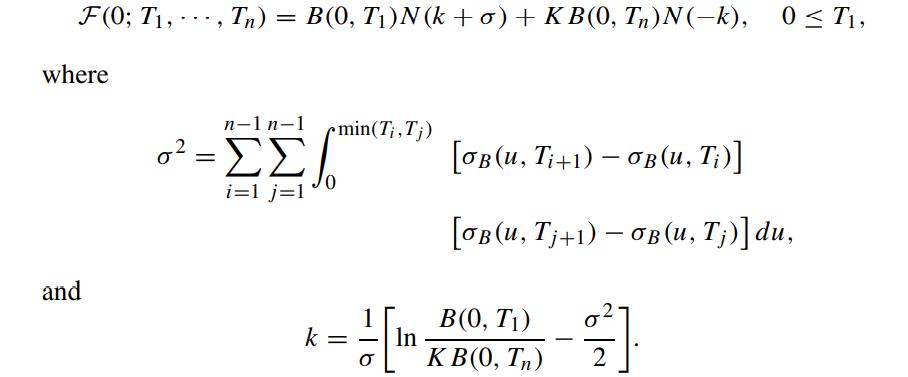Problem 8.21

Assume that the T-maturity discounted bond price process B(t,T) follows the one-factor Gaussian HJM under the risk neutral measure Q:

A caption is a call option on a cap, whose terminal payoff at time T is given by

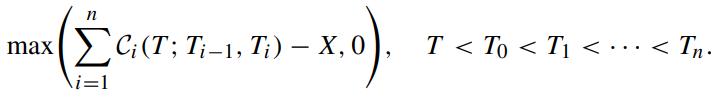
Here, Ci(T ; T_i−1,T_i) is the time-T value of a caplet with payment on the LIBOR L_i−1 at time T_i and X is the strike price, i = 1, 2, ··· ,n. Since a cap can be visualized as a series of put options on the zero-coupon bonds, a caption is seen as a compound call on a put. By applying Jamshidian’s decomposition technique (Jamshidian, 1989) for an option on a coupon-bearing bond, find the time-t value of the caption, t Use the identity