Consider the multifactor extension of the InuiKijima model. Let i F (t, T ), i =

Consider the multifactor extension of the Inui–Kijima model. Let σⁱ_F (t, T ), i = 1, 2, ··· ,n, satisfy

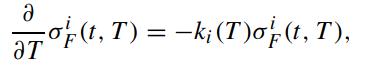for some deterministic function k_i(T ) and initial condition

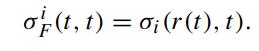
Define 

where 

Also, show that (i) it is possible to express one of ψ_i(t) in terms of r(t) and remaining ψ_i(t), that is, the process

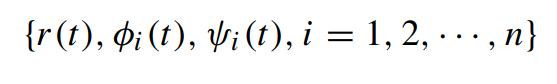forms a 2n-dimensional Markov system; and (ii) r(t) is mean-reverting.

Transcribed Image Text:

Ә ar o+(t,T) = -k;(T)o}(t, T), aT