Frullani's integral. Let f : ( 0 , ) R f : ( 0 ,

Frullani's integral. Let $f:(0,\infty )\to R$ be a continuous function such that ${lim}_{x\to 0}f\left(x\right)=m$ and ${lim}_{x\to \infty }f\left(x\right)=M$. Show that the two-sided improper Riemann integral

$$\underset{\begin{array}{c}r\to 0\\ s\to \infty \end{array}}{lim}{\int }_r^s\frac{f\left(bx\right)-f\left(ax\right)}{x}dx=(M-m)\ln \left(\frac{b}{a}\right)$$

exists for all $a,b>0$. Does this integral have a meaning as a Lebesgue integral?

[ use the mean value theorem for integrals, Corollary I.12.]

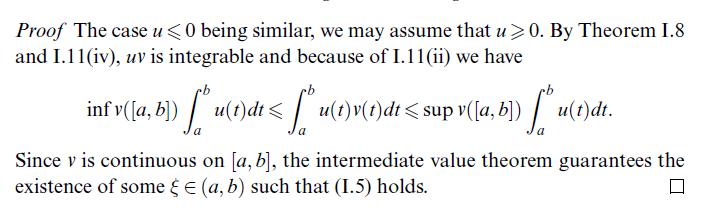
Data from corollary I.12

Transcribed Image Text:

(mean value theorem for integrals) Let u R[a, b] be either positive or negative and let ve C[a, b]. Then there exists some & E (a, b) such that f* u(t)v(1)dt = v(s) ["* u(1)di. a (1.5)