Let \((X, \mathscr{A}, \mu)\) be a \(\sigma\)-finite measure space and assume that \(\mathscr{A}\) is generated by a Boolean algebra \(\mathscr{G}\), i.e. a family of subsets of \(X\) which is stable under finite unions, intersections and complementation and contains \(\emptyset\). Show that every \(u \in \mathcal{M}(\mathscr{A})\) can be approximated.

by a sequence \(\left(f_{n}ight)_{n \in \mathbb{N}} \subset \mathcal{E}(\mathscr{G})\) such that \(\lim _{n ightarrow \infty} f_{n}(x)=u(x)\) for all \(x \in N^{c}\), where \(N \in \mathscr{A}\) has measure zero: \(\mu(N)=0\). (The set \(N\) may, and will in general, depend on \(u\) ).

[ use the result from Problem 5.12 and combine it with Theorem 8.8 or Theorem 8.15 .]

Remark. If \(\mu\) is Lebesgue measure on \(X=\mathbb{R}, \mathscr{A}=\mathscr{B}(\mathbb{R})\), we can use \(\mathscr{G}\) as the family of finite unions of all half-open intervals \([a, b),-\infty \leqslant a \leqslant b \leqslant+\infty\). It is not hard to see that this is a Boolean algebra. The problem tells us that we can approximate any measurable function up to a null set(!) by step-functions (with finitely many steps) with basis sets of the form \([a, b)\). You should compare this result with the comparison results between the Lebesgue and Riemann integrals, see Chapter 12: in some sense this leads to a Riemannsum approximation of a Lebesgue integral.

Data from problem 5.12

Data from theorem 8.8

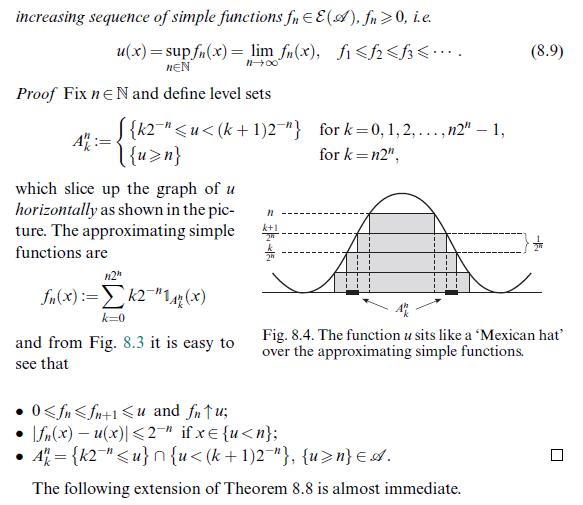
Data from theorem 8.15

Transcribed Image Text:

Approximation of o-algebras. Let be a Boolean algebra in X, i.e. a family of sets such that XE and is stable under the formation of finite unions, intersections and complements. Let = o(9) and be a finite measure on (X, A, ). Let A B:= (A\B)U(B\A) denote the symmetric difference of A, BCX. (i) For every > 0 and A there is some Gesuch that (4 AG) < . [Hint: {AE:Ve> 0G9(AG) e} is a Dynkin system.] (ii) Let u, v be finite measures on (X, ). For every >0 and A there is some GEG such that (AAG) e and v(AAG) < E. (iii) Assume that X=R", A = B(R") and u=X". A set AE satisfies (4) = 0 if, and only if, for every >0 there is a sequence (In)nEN CJ, such that ACU, In and (U, In) E. Remark. Compare the result of this exercise with Theorem 27.25.