Show that \(x \mapsto \max \{x, 0\}\) and \(x \mapsto \min \{x, 0\}\) are continuous, and by virtue of Problem 8.7 or Example 7.3 , measurable functions from \(\mathbb{R} ightarrow \mathbb{R}\). Conclude that on any measurable space \((X, \mathscr{A})\) positive and negative parts \(u^{ \pm}\)of a measurable function \(u: X ightarrow \mathbb{R}\) are measurable.

Data from problem 8.7

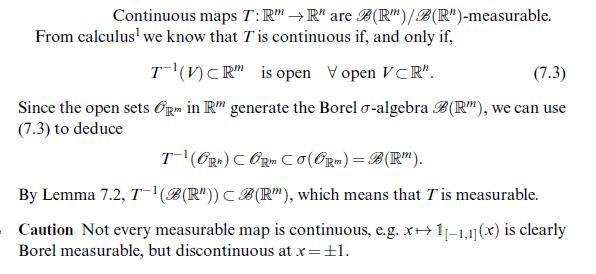
Show that every continuous function \(u: \mathbb{R} ightarrow \mathbb{R}\) is \(\mathscr{B}(\mathbb{R}) / \mathscr{B}(\mathbb{R})\)-measurable.

[ check that for continuous functions \(\{u>\alpha\}\) is an open set.]

Data from example 7.3

Transcribed Image Text:

Continuous maps 7: RR" are (R")/B(R")-measurable. From calculus we know that I' is continuous if, and only if, T(V) CR is open V open VCR". (7.3) Since the open sets OR in R" generate the Borel o-algebra B(R"), we can use (7.3) to deduce T- (OR) CORM CO (OR)= B(R"). By Lemma 7.2, T- (B(R")) CB(R"), which means that T is measurable. Caution Not every measurable map is continuous, e.g. x+11-1,1] (x) is clearly Borel measurable, but discontinuous at x = 1.