A body of mass (m=11.0 mathrm{~g}) can move by resting on a rail, which is frictionless, that
Question:
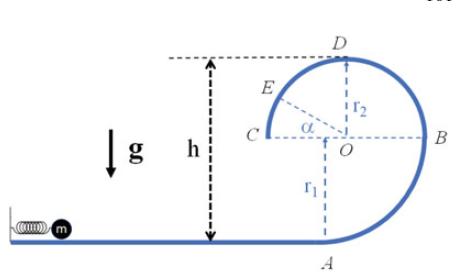
A body of mass \(m=11.0 \mathrm{~g}\) can move by resting on a rail, which is frictionless, that runs in the vertical plane with the shape shown in the Fig.6.8
: a horizontal section to A, a quarter circle of radius \(r_{1}\) from point \(\mathrm{A}\) to point \(\mathrm{B}\), a semicircle of radius \(r_{2}\) from point \(\mathrm{B}\) to point C. The body is initially at rest on the free end of a spring attached at the other end to the rail. The spring, of elastic constant \(k=1.73 \mathrm{~N} / \mathrm{m}\), is initially compressed by \(\Delta x=38.0 \mathrm{~cm}\). Knowing that \(h=r_{1}+r_{2}=100 \mathrm{~cm}\), determine:
1. What is the maximum value that the radius \(r_{2}\) can take for the body to reach point \(\mathrm{D}\) without losing contact with the rail. For this value of \(r_{2}\) calculate:
2. the velocity of the body as it passes through point B;
3. the speed at point \(\mathrm{E}\), knowing that the angle formed by the segment \(\overline{O E}\) with the horizontal \(O C\) is \(\alpha=\pi / 6\).
4. the magnitude and direction of the constraining reaction, applied by the guide to the body of mass \(m\), at point \(\mathrm{E}\).
Fig. 6.8
Step by Step Answer:






