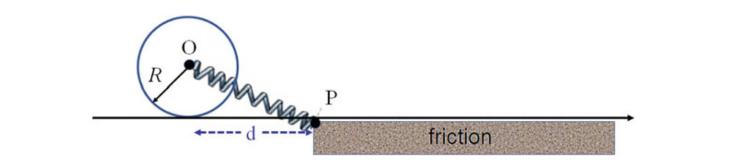
A cylinder of mass \(M=2.0 \mathrm{~kg}\) and radius \(R=10 \mathrm{~cm}\) is resting on a horizontal plane, and its center \(\mathrm{O}\) is connected to a point \(\mathrm{P}\) on the plane by a spring (with negligible length at rest) of spring constant \(k=150 \mathrm{~N} / \mathrm{m}\), see Fig. 12.15. The cylinder is initially stationary, its axis is perpendicular to the sheet, and the resting point of the cylinder is to the left of \(\mathrm{P}\), at a distance \(d=40 \mathrm{~cm}\) from it. In the region to the left of point \(\mathrm{P}\) there is no friction; to the right of point \(\mathrm{P}\) the plane is rough and the cylinder is constrained to roll without crawling. Determine:

1. The velocity of the center of mass of the cylinder when the point of contact arrives at \(\mathrm{P}\) (immediately before).

2. The moment of inertia of the cylinder with respect to an axis perpendicular to the sheet passes through the point \(P\), when the point of contact is in \(P\).

3. the angular speed of the cylinder immediately after the point of contact arrives at P;

4. the maximum distance, \(d_{A}\), traveled by the contact point of the cylinder in the region with friction immediately to the right of the point \(\mathrm{P}\).

(Note: The cylinder always moves while keeping its axis parallel to itself.)

 Fig. 12.15

Transcribed Image Text:

R d P friction