Consider two rods of equal length (L=17.2 mathrm{~cm}) and masses (M) and (3 M) (with (M=153 mathrm{~g})
Question:
Consider two rods of equal length \(L=17.2 \mathrm{~cm}\) and masses \(M\) and \(3 M\) (with \(M=153 \mathrm{~g}\) ) glued to each other as in Fig. 12.16. The system of the two rods is constrained to rotate (with negligible friction) about an axis passing through an extreme \(O\). Initially, the two-rod system is stationary in the stable equilibrium position. A mass \(m=M / 2\) impacts the system at point \(D\) at a distance \(h=7 / 4 L\) from the axis of rotation. The velocity of \(m\) before the collision has magnitude \(v_{0}=3.70 \mathrm{~m} / \mathrm{s}\) and forms an angle \(\theta=45^{\circ}\) with the perpendicular to the rod. After the impact, the mass remains embedded in the rod. Calculate:
1. the moment of inertia \(J\) of the system with respect to the axis of rotation \(O\);
2. the distance \(d\) of the center of mass of the system from the axis of rotation;
3. the angular speed \(\omega\) of the system after impact;
4. the horizontal and vertical components of the impulse due to the constraining force that the axis of rotation applies to the bar, which is necessary to conserve linear momentum.
Fig. 12.16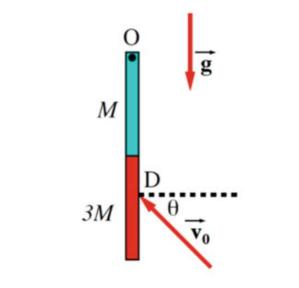
Step by Step Answer:






