(a) Integrate Langevin's equation (15.3.5) for the velocity component \(v_{x}\) over a small interval of time \(\delta t\), and show that

Equation (15.3.5)

.\[
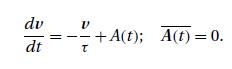
\frac{\left\langle\delta v_{x}\rightangle}{\delta t}=-\frac{v_{x}}{\tau} \quad \text { and } \quad \frac{\left\langle\left(\delta v_{x}\right)^{2}\rightangle}{\delta t}=\frac{2 k T}{M \tau}
\]
(b) Now, set up the Fokker-Planck equation for the distribution function \(f\left(u_{x}, t\right)\) and, making use of the foregoing results for \(\mu_{1}\left(v_{x}\right)\) and \(\mu_{2}\left(v_{x}\right)\), derive an explicit expression for this function. Study the various cases of interest, especially the one for which \(t \gg \tau\).

dv V +A(t); A(t)=0. dt