The invariance of transverse lengths. A stick of length (L_{0}) is placed at rest along the (y^{prime})
Question:
The invariance of transverse lengths. A stick of length \(L_{0}\) is placed at rest along the \(y^{\prime}\) axis of the primed frame, extending from \(y^{\prime}=y_{1}^{\prime}\) to \(y^{\prime}=y_{2}^{\prime}\). Observers in the unprimed frame measure the position of both ends of the stick at the same time \(t\) to them as the stick is moving along at speed \(V\). Using the Lorentz transformation of Eqs. 2.15, find the length \(L \equiv\left(y_{2}-y_{1} \right)\) of the stick in the unprimed frame, in terms of \(L_{0}\) and the relative frame velocity \(V\). Here \(y_{2}\) and \(y_{1}\) are the locations of each end of the stick, as measured in the unprimed frame. The fact that \(L=L_{0}\) is an example of the fact that moving transverse lengths are invariant under Lorentz transformations.
Data from Eqs. 2.15
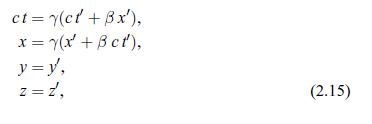
Step by Step Answer:






