An owner of a strawberry plantation hires one worker who has 24 units of time. The employee can allocate part of the time to work and part to rest. He/she owns 20% of shares in profits of the plantation. The only production factor in producing strawberries is the labour of the worker. The process of production of strawberries is described by a power production function of a form: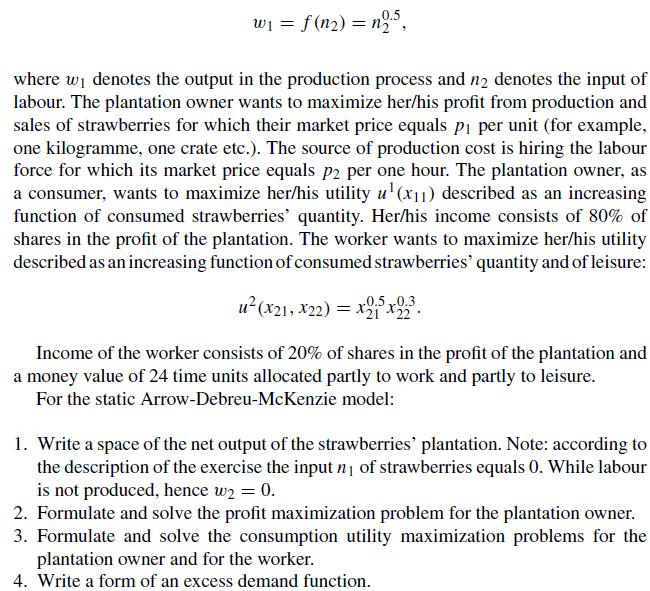

Transcribed Image Text:

w = f(n) = no.5, where w denotes the output in the production process and no denotes the input of labour. The plantation owner wants to maximize her/his profit from production and sales of strawberries for which their market price equals p per unit (for example, one kilogramme, one crate etc.). The source of production cost is hiring the labour force for which its market price equals p2 per one hour. The plantation owner, as a consumer, wants to maximize her/his utility u (11) described as an increasing function of consumed strawberries' quantity. Her/his income consists of 80% of shares in the profit of the plantation. The worker wants to maximize her/his utility described as an increasing function of consumed strawberries' quantity and of leisure: 0.3 u(x21, x22) = x215x22. Income of the worker consists of 20% of shares in the profit of the plantation and a money value of 24 time units allocated partly to work and partly to leisure. For the static Arrow-Debreu-McKenzie model: 1. Write a space of the net output of the strawberries' plantation. Note: according to the description of the exercise the input n of strawberries equals 0. While labour is not produced, hence w = 0. 2. Formulate and solve the profit maximization problem for the plantation owner. 3. Formulate and solve the consumption utility maximization problems for the plantation owner and for the worker. 4. Write a form of an excess demand function.