(a) Consider the Penrose process, described in the text, in which a particle flying inward toward a...
Question:
(a) Consider the Penrose process, described in the text, in which a particle flying inward toward a spinning hole’s horizon splits in two inside the ergosphere, and one piece plunges into the hole while the other flies back out. Show that it is always possible to arrange this process so the plunging particle has negative energy at infinity:
![]()
(b) Around a spinning black hole consider the vector field
![]()
where ΩH is the horizon’s angular velocity. Show that at the horizon (at radius r = rH) this vector field is null and is tangent to the horizon generators. Show that all other vectors in the horizon are spacelike.
(c) In the Penrose process the plunging particle changes the hole’s mass by an amount △M and its spin angular momentum by an amount △JH. Show that
![]()
Here
![]()
are to be evaluated at the event where the particle plunges through the horizon, so they both reside in the same tangent space.
(d) Show that if A(vector) is any future-directed time like vector and K(vector) is any null vector, both living in the tangent space at the same event in spacetime, then A(vector) · K(vector)
![]()
is positive, whatever may be the world line and rest mass of the plunging particle.
(e) Show that for the plunging particle to decrease the hole’s mass, it must also decrease the hole’s angular momentum (i.e., it must spin the hole down a bit).
(f) Hawking’s second law of black-hole mechanics says that, whatever may be the particle’s world line and rest mass, when the particle plunges through the horizon, it causes the horizon’s surface area AH to increase. This suggests that the always positive quantity
![]()
might be a multiple of the increase △AH of the horizon area. Show that this is indeed the case:
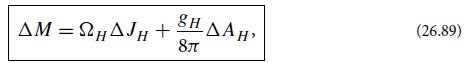
where gH is given in terms of the hole’s mass M and the radius rH of its horizon by

The quantity gH is called the hole’s “surface gravity” for a variety of reasons. One reason is that an observer who hovers just above a horizon generator, blasting his or her rocket engines to avoid falling into the hole, has a 4-acceleration with magnitude gH/α and thus feels a “gravitational acceleration” of this magnitude; here α = gtt is a component of the Kerr metric called the lapse function [Eqs. (26.70) and (26.72)]. This gravitational acceleration is arbitrarily large for an observer arbitrarily close to the horizon (where △ and hence α are arbitrarily close to zero); when renormalized by α to make it finite, the acceleration is gH. Equation (26.89) is called the “first law of black-hole mechanics” because of its resemblance to the first law of thermodynamics.
(g) Stephen Hawking has shown, using quantum field theory, that a black hole’s horizon emits thermal (blackbody) radiation. The temperature of this “Hawking radiation,” as measured by the observer who hovers just above the horizon, is proportional to the gravitational acceleration gH/α that the observer measures, with a proportionality constant ℏ/(2πkB), where ℏ is Planck’s reduced constant and kB is Boltzmann’s constant. As this thermal radiation climbs out of the horizon’s vicinity and flies off to large radii, its frequencies and temperature get redshifted by the factor α, so as measured by distant observers the temperature is

This suggests a reinterpretation of the first law of black-hole mechanics (26.89) as the first law of thermodynamics for a black hole:
![]()
where SH is the hole’s entropy [cf. Eq. (4.62)]. Show that this entropy is related to the horizon’s surface area by

where
![]()
is the Planck length (with G Newton’s gravitation constant and c the speed of light). Because SH ∝ AH, the second law of black-hole mechanics (nondecreasing AH) is actually the second law of thermodynamics (nondecreasing SH) in disguise.6
(h) For a 10-solar-mass, nonspinning black hole, what is the temperature of the Hawking radiation in Kelvins, and what is the hole’s entropy in units of the Boltzmann constant?
(i) Reread the discussions of black-hole thermodynamics and entropy in the expanding universe in Secs. 4.10.2 and 4.10.3, which rely on the results of this exercise.
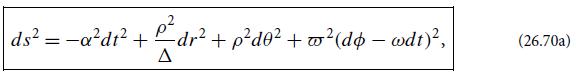
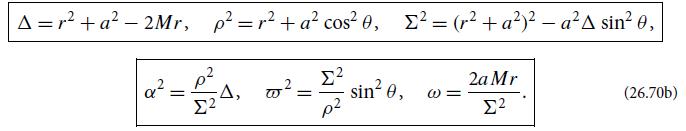
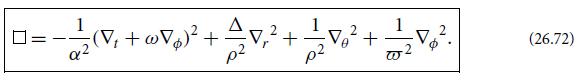
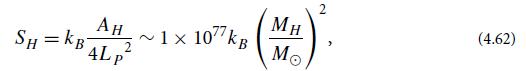
Step by Step Answer:

Modern Classical Physics Optics Fluids Plasmas Elasticity Relativity And Statistical Physics
ISBN: 9780691159027
1st Edition
Authors: Kip S. Thorne, Roger D. Blandford





