A narrow bundle of magnetic field lines with cross sectional area A, together with the plasma attached to them, can be thought of as like a stretched string. When such a string is plucked, waves travel down it with phase speed √T/∧, where T is the string’s tension. The plasma analog is Alfvén waves propagating parallel to the plasma-laden magnetic field.

(a) Analyzed nonrelativistically, the tension for our bundle of field lines is T = [B²/(2μ₀)]A and the mass per unit length is ∧ = ρA, so we expect a phase velocity

which is 1/√2 of the correct result. Where is the error?

(b) In special relativity, the plasma-laden magnetic field has a tensorial inertial mass per unit volume that is discussed in Ex. 2.27. Explain why, when the field lines (which point in the z direction) are plucked so they vibrate in the x direction, the inertial mass per unit length that resists this motion is ∧ = (T⁰⁰ + T^xx)A = [ρ + B²/(μ₀c²)]A. Show that the magnetic contribution to this inertial mass gives the relativistic correction

to the Alfvén waves’ phase speed, Eq. (21.56).

Equation 21.56.

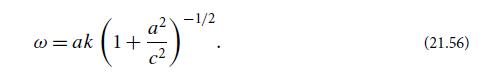
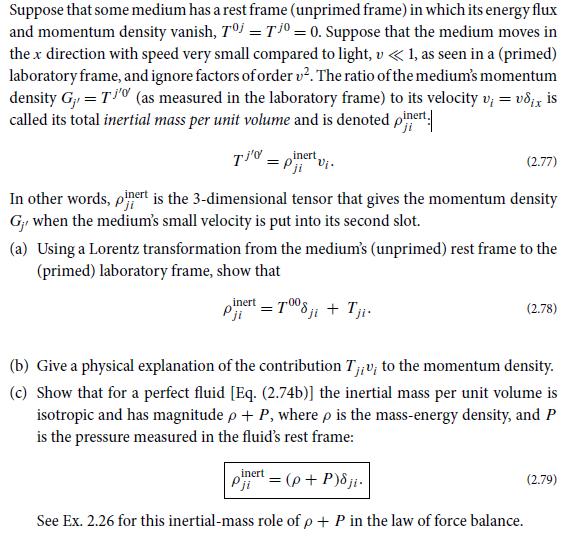
Data from Exercises 2.27.

Transcribed Image Text:

(Τ/Λ = |B2/(2μορ),