Consider the three-wave processes shown in Fig. 23.5, with A and C Langmuir plasmons and B an ion-acoustic plasmon. The fundamental rate is given by Eqs. (23.56a) and (23.56b).

(a) By summing the rates of forward and backward reactions (Fig. 23.5a,b), show that the occupation number for the ion-acoustic plasmons satisfies the kinetic equation

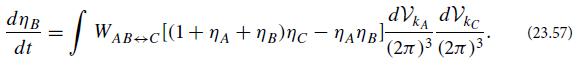
(b) The ion-acoustic plasmons have far lower frequencies than the Langmuir plasmons, so ω_B ≪ ω_A ≈ ω_C. Assume that they also have far lower wave numbers, |k_B| ≪ |k_A| ≈ |k_C|. Assume further (as will typically be the case) that the ionacoustic plasmons, because of their tiny individual energies, have far larger occupation numbers than the Langmuir plasmons, so η_B ≫ η_A ∼ η_C. Using these approximations, show that the evolution law (23.57) for the ion-acoustic waves reduces to the form

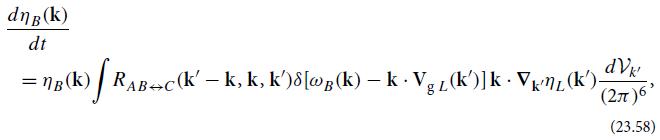
where η_L is the Langmuir (waves A and C) occupation number, V_{g L} is the Langmuir group velocity, and R_C↔BA is the fundamental rate (23.56b).

(c) Notice the strong similarities between the evolution equation (23.58) for the ion-acoustic plasmons that are Cerenkov emitted and absorbed by Langmuir plasmons, and the evolution equation (23.44) for Langmuir plasmons that are Cerenkov emitted and absorbed by fast electrons! Discuss the similarities and the physical reasons for them.

(d) Challenge: Carry out an explicit classical calculation of the nonlinear interaction between Langmuir waves with wave vectors k_A and k_C to produce ion-acoustic waves with wave vector k_B = k_C − k_A. Base your calculation on the nonlinear Vlasov equation (23.55) and [for use in relating E and f₁ in the nonlinear term]the 3-dimensional analog of Eq. (23.13). Assume a spatially independent Maxwellian averaged electron velocity distribution f₀ with temperature T_e (so ∇f₀ = 0). From your result compute, in the random-phase approximation, the evolution of the ion-acoustic energy density ε_k and thence the evolution of the occupation number η(k). Bring that evolution equation into the functional form (23.58). By comparing quantitatively with Eq. (23.58), read off the fundamental rate R_C↔BA. Your result should be the rate in Eq. (23.56b).

Equations:

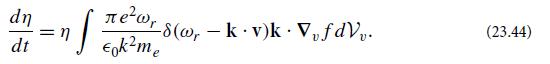
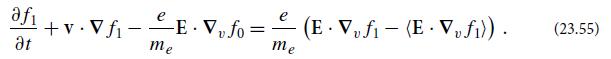
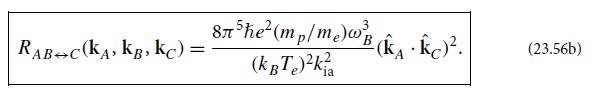
Figure 23.5.

Transcribed Image Text:

dn B dt = = √ WAB+c[(1+NA+NB)nc - NAMB dVkA dVkc (2π)³ (2π)³ (23.57)