Exercise 5.5 explored the enthalpy representation of thermodynamics for an equilibrium ensemble of systems in contact with a volume bath. Here we extend that analysis to an ensemble out of equilibrium. We denote by P_b the bath pressure.

(a) The systems exchange volume but not heat or particles with the bath. Explain why, even though the ensemble may be far from equilibrium, any system’s volume change dV must be accompanied by an energy change dε = −P_bdV . This implies that the system’s enthalpy H = ε + P_bV is conserved. All systems in the ensemble are assumed to have the same enthalpy H and the same number of particles N.

(b) Using equilibrium considerations for the bath, show that interaction with a system cannot change the bath’s entropy.

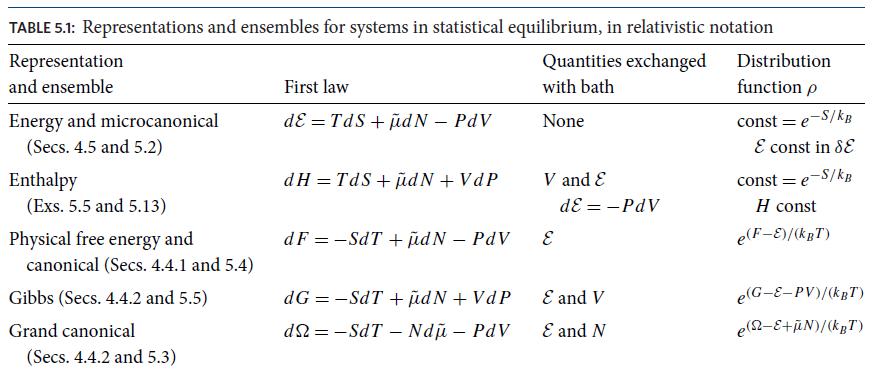
(c) Show that the ensemble will always evolve toward increasing entropy S, and that when the ensemble finally reaches statistical equilibrium with the bath, its distribution function will be that of the enthalpy ensemble (Table 5.1): ρ = e^−S/kB = const for all regions of phase space that have the specified particle number N and enthalpy H.

(d) Show that fluctuations away from equilibrium are described by the probability distributions (5.69a) and (5.69c), but with the system energy E replaced by the system enthalpy H and the system volume V replaced by the bath pressure P_b.

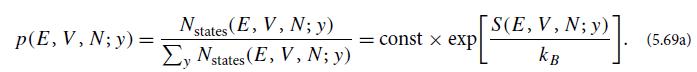
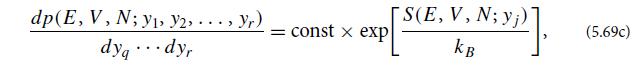
Table 5.1

Data from Exercises 5.5

(a) Enthalpy H is a macroscopic thermodynamic variable defined by

Show that this definition can be regarded as a Legendre transformation that converts from the energy representation of thermodynamics with E(V , S, N) as the fundamental potential, to an enthalpy representation with H(P , S, N) as the fundamental potential. More specifically, show that the first law, re-expressed in terms of H, takes the form

and then explain why this first law dictates that H(P , S, N) be taken as the fundamental potential.

(b) For a nonrelativistic system, it is conventional to remove the particle rest masses from the enthalpy just as one does from the energy, but by contrast with energy, we do not change notation for the enthalpy:

What is the form of the first law (5.47) for the nonrelativistic H?

(c) There is an equilibrium statistical mechanics ensemble associated with the enthalpy representation. Show that each system of this ensemble (fluctuationally) exchanges volume and energy with a surrounding pressure bath but does not exchange heat or particles, so the exchanged energy is solely that associated with the exchanged volume, dE = −PdV, and the enthalpy H does not fluctuate. Note that P is the common pressure of the bath and the system.

(d) Show that this ensemble’s distribution function is ρ = e^−S/kB = constant for those states in phase space that have a specified number of particles N and aspecified enthalpy H. Why do we not need to allow for a small range δH of H, by analogy with the small range E for the microcanonical ensemble.

(e) What equations of state can be read off from the enthalpy first law? What are the Maxwell relations between these equations of state?

(f) What is the Euler equation for H in terms of a sum of products of extensive and intensive variables?

(g) Show that the system’s enthalpy is equal to its total (relativistic) inertial mass (multiplied by the speed of light squared);

(h) As another interpretation of the enthalpy, think of the system as enclosed in an impermeable box of volume V . Inject into the box a “sample” of additional material of the same sort as is already there. (It may be helpful to think of the material as a gas.) The sample is to be put into the same thermodynamic state (i.e.,macrostate) as that of the box’s material (i.e., it is to be given the same values of temperature T , pressure P, and chemical potential μ̃). Thus, the sample’s material is indistinguishable in its thermodynamic properties from the material already in the box, except that its extensive variables (denoted by △) arefar smaller:

Perform the injection by opening up a hole in one of the box’s walls, pushing aside the box’s material to make a little cavity of volume △V equal to that of the sample, inserting the sample into the cavity, and then closing the hole in the wall. The box now has the same volume V as before, but its energy has changed. Show that the energy change (i.e., the energy required to create the sample and perform the injection) is equal to the enthalpy △H of the sample. Thus, enthalpy has the physical interpretation of energy of injection at fixed volume V . Equivalently, if a sample of material is ejected from the system, the total energy that will come out (including the work done on the sample by the system during the ejection) is the sample’s enthalpy △H. From this viewpoint, enthalpy is the system’s free energy.

Transcribed Image Text:

p(E, V, N; y) = Nstates (E, V, N; y) Ey Nstates (E, V, N; y) = const x exp N;)]. S(E, V, N; y) KB (5.69a)