A charge Q is distributed uniformly on a non-conducting ring of radius R and mass M. The ring is dropped from rest from a height h and falls to the ground through a non-uniform magnetic field B(r). The plane of the ring remains horizontal during its fall.
(a) Explain qualitatively why the ring rotates as it falls.
(b) Use Faraday’s flux rule to show that the velocity of the center of mass of the ring when it hits the ground is

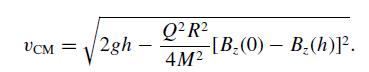
(c) The magnetic Lorentz force in this problem plays the role of static friction when a massive cylinder rolls down a rough incline. Both facilitate the transfer of translational kinetic energy into rotational kinetic energy without performing net work themselves. Thus the rotation in part (a) may be ascribed to the work done by an azimuthal Lorentz force associated with the vertical component of the ring velocity and the horizontal components of the magnetic field. If so, an equal and opposite magnetic work must be done by a vertical Lorentz force which opposes the force of gravity. Confirm that this is the case by an explicit calculation of this force and the net work it performs on the ring. Assume that the magnetic field changes slowly over the area of the ring at any time during its fall.

Transcribed Image Text:

UCM 2gh Q²R² 4M² -[B₂(0) B₂(h)]².