Question: A periodic function f(t) of period 2 is defined within the period 0 t 2 by. Draw a graph of the function for

A periodic function f(t) of period 2π is defined within the period 0 ≤ t ≤ 2 by.

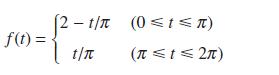
f(t) = -t/ t/ (0 t ) ( t 2)

Draw a graph of the function for –4π ≤ t ≤ 4π and obtain its Fourier series expansion. By replacing t by t – 1/2π in your answer, show that the periodic function

is represented by a sine series of odd harmonics.

f(t) = -t/ t/ (0 t ) ( t 2)

Step by Step Solution

★★★★★

3.35 Rating (155 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

4R ao I 3 2t In I 1 27 2 12 sinnt I n 4 22 n 2x t K I 2x 1 E... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Modern Engineering Mathematics Questions!

There is a 120V 40 W light bulb, which statement is INCORRECT? Othe rate of energy dissipation is 40 W the current flowing through is 0.33 A the capacitance is 3.0 Farad O the light bulb will...

A periodic function f(t) of period 2 is defined within the domain 0 t by Sketch a graph of f(t) for 2 (a) f(t) is an even function; (b) f(t) is an odd function. Find the Fourier series expansion...

A periodic function f(t) of period 2 is defined in the interval 1

Asif Trading Ltd was established during 2017 with an authorized capital of 200,000 common stock of Rs. 50 each. The Company issued 150,000 Common Stock to the public at Rs. 60 each during August,...

Consider the trigonometric series a0 2 + X r=1 (ar cos rx + br sin rx) where a0, a1, a2, . . . and b1, b2, . . . are constants and suppose that f(x) is a periodic function of x with period 2. (a)...

1) State the initial value theorem. Verify the theorem for the functions (a) 3 4 sin t (b) (t 4)2 and state their initial values. [(a) 3 (b) 16] 2. Verify the initial value theorem for the voltage...

MAT1510/101 ASSIGNMENT 03 Due Date: 17 June 2016 Total Marks: 100 UNIQUE ASSIGNMENT NUMBER: 736826 ONLY FOR YEAR MODULE STUDY MATERIAL: SRW and STUDY GUIDE: CHAPTER 4 The answers to only some of the...

question 1 The functions q and r are defined as follows. q (x) = -5x+4 r (x) =-4x+4 Find the value of q (r (-2)). 9 (x (-2)) = 0 X 5 ?Suppose that the functions q and y are defined as follows. q (x)...

1. The function f graphed below is defined by a polynomial expression of degree 4. Use the graph to solve the exercise. 3 (a) A function value f(a) is a local maximum value of fif f(a) is the...

(2) Consider the periodic function defined within one period by the formula: w ( x ) =x+ 5 x+- if - -Sxs- (a) . Simplify this function and plot it on the interval -2.5 .x $ 2.5. Determine whether the...

question 1 A function is defined over (0, 2) by We then extend it to an even periodic function of period 4 -4 -2 and its graph is displayed below. -6 2 15 05 -05 2 4 6 8 The function may be...

On March 1, Sheridan Corp. issues a 3 year, 7%, $54000 bond at face value. Interest is paid semi-annually. The entry to record the first interest payment will include a O debit to Cash of $1890....

Harmony Forge manufactures saddles for show horses. The company has received a special order for 290 saddles for an international competition. Each of these saddles would include the specialized logo...

Actuation Consulting and Enterprise Agility recently conducted a global survey of product teams with the goal of better understanding the dynamics of product team performance and uncovering the...

3. How can marketers reassure consumers about privacy concerns if RFID comes into widespread use? As mentioned in the chapter, some analysts predict bar codes may soon be replaced by a wireless...

The specific gravity of benzene is 0.876. Calculate its specific weight and its density in SI units.

A cylindrical can 150 mm in diameter is filled to a depth of 100 mm with a fuel oil. The oil has a mass of 1.56 kg. Calculate its density, specific weight, and specific gravity.

Glycerin has a specific gravity of 1.258. How much would 0.50 m 3 of glycerin weigh? What would be its mass?

Alpha Company made a lump sum purchase of land, building, and equipment. The following were the appraised values of each element: Land - $10,000 Building - $20,000 Equipment - $30,000 Alpha paid...

Baker had the following normal account balances just prior to transferring them to a new partnership Cash $12,000, Inventory $35,000Equipment $31,000Accumulated Depreciation $15,000 Assuming the fair...

The neighbour that you have been renting land from for the last 5 years has decided to sell you the section of land (640 ac) for $2,000 per acre. Answer the following: Be sure to show your work. If...