it was stated that a symmetric matrix A has real eigenvalues 1 , 2 ,

it was stated that a symmetric matrix A has real eigenvalues λ₁, λ₂, . . . . , λ_n (written in descending order) and corresponding orthonormal eigenvectors e₁, e₂, . . . . , e_n, that is e^T_ie_j= δ_ij. In consequence any vector can be written as

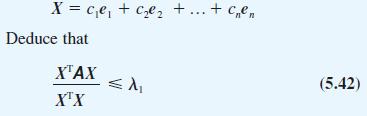
so that a lower bound of the largest eigenvalue has been found. The left-hand side of (5.42) is called the Rayleigh quotient. It is known that the matrix

has a largest eigenvalue of 1/2((1 + √5)). Check that the result (5.42) holds for any vector of your choice.