(a) Show that the most general \(2 \times 2\) unitary matrix with unit determinant can be parameterized as in Eqs. (6.76) and (6.77). 

(b) Take the group identity element \(U(1,0,0,0)\) to correspond to \(r_{1}=r_{2}=r_{3}=0\) and expand around the identity to show that \(U \simeq 1-i d r_{i} \sigma_{i}\), where \(\sigma_{i}\) is a Pauli matrix.

Data from Eq. 6.76

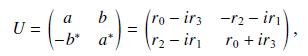
Data from Eq. 6.77

Transcribed Image Text:

b (ro-ir3 -r2-in 1 = = (-2)-(2-22-7). -b* a* + ir